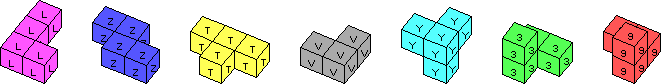
L	Z	T	V	Y	3	9

Because convex shapes are excluded, the 2 x 2 square and the 1 x 4 row of cubelets are not part of the Soma set. These seven pieces can be assembled to form the 3 x 3 x 3 Soma cube, as well as thousands of other designs.

In order to distinguish these pieces from each other, we can use a simple naming convention based on letters of the alphabet. Most of the pieces suggest obvious choices. For the last two, we've chosen names based on a clock, which usually has its minute hand in front of its hour hand. The 3 piece suggests the appearance of the hands at 3 o'clock: the minute hand is in front pointing upward, and the hour hand is in back pointing to the right. Similarly, the 9 piece corresponds to a front clock hand pointing upward and a back hand pointing to the left ("9 o'clock"). In addition to this notation, our solutions show each Soma piece uniquely colored, with each of its visible faces labeled with the piece name.

The majority of the designs shown on these pages can be constructed with a single 7-piece Soma set (27 cubelets), but some puzzles are larger designs made from two or more Soma sets. Multiple solutions are often possible for many of the designs. A section of this site is devoted to Shrinking Somas, which are special puzzles involving Soma pieces placed in an enclosing box.

Notation

The dimensions of a Soma design will be denoted by R rows and C columns, often with R ≤ C, and a height of L levels, oriented as in the following diagram:

Convexity

A convex three-dimensional shape is one that has no raised cubelets, dents, interior corners or holes (visible or hidden). All of its corners are exterior. Formally, convexity requires that the line connecting any two points inside the shape is completely inside it. It is therefore evident that the only convex shape that can be created from a set of identical cubelets is a cuboid, or box shape.

The only convex shape that can be created from exactly one Soma set is the Soma cube itself. The non-flat pieces force the cuboid dimensions R, C and L to all be at least 2; in other words, a flat slab cannot be created from all 7 of the Soma pieces. Since the product RCL = 27, the only possible solution is R = C = L = 3. Larger cuboids can be created from multiple Soma sets by arranging individual 3 x 3 x 3 volumes in a row, a rectangle or a cube. The only other one that can be created from two Soma sets is the 2 x 3 x 9 cuboid.

Use the Interactive Soma Machine to create your own Soma designs!