Balmoral Software
Balmoral Software

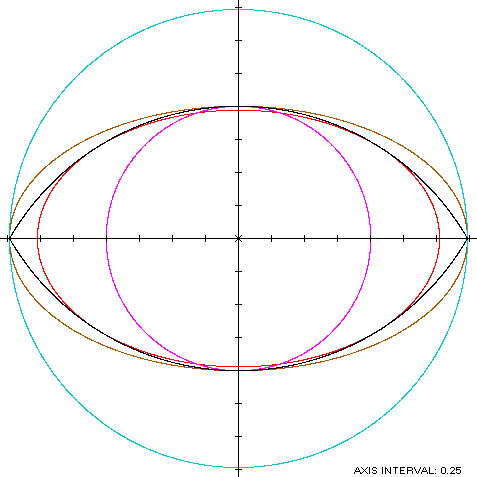
The abscissa extrema of S are at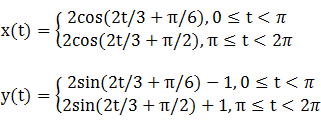
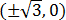 and the ordinate extrema at the
two circle centers (0,±1), so the width x height of the bounding
rectangle of S is
and the ordinate extrema at the
two circle centers (0,±1), so the width x height of the bounding
rectangle of S is  .
.
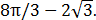
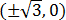 , so the inradius is 1 and the
circumradius is
, so the inradius is 1 and the
circumradius is 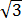 . Since these
extrema occur on the coordinate axes, a candidate for the circumellipse is one
enclosed by the annulus between the two boundary circles, with
. Since these
extrema occur on the coordinate axes, a candidate for the circumellipse is one
enclosed by the annulus between the two boundary circles, with
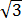
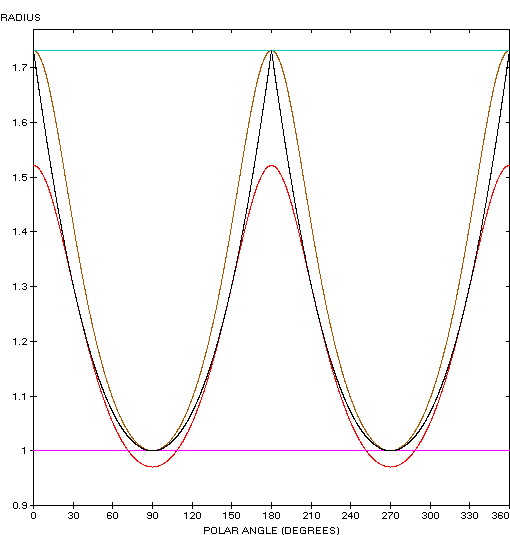
x(t)y(t) = 2cos(2t/3 + π/6)[2sin(2t/3 + π/6) - 1]This expression is maximized in the first quadrant at the complicated value
The circumellipse dimensions are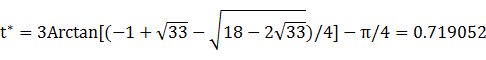
For verification, we have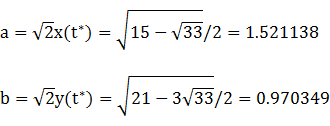
Figure Parameters Perimeter Area Centroid Incircle R = 1 6.283185 3.141593 Inellipse 7.923165 4.637100 Vesica Piscis 8.377580 4.913479 Circumellipse 8.737753 5.441399 Circumcircle R = 10.882796 9.424778
 |  |
| "The CBS Television Network" |
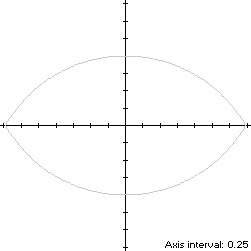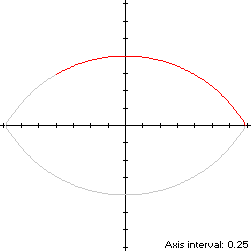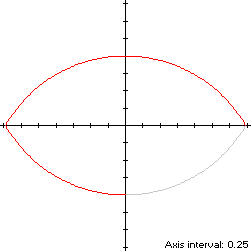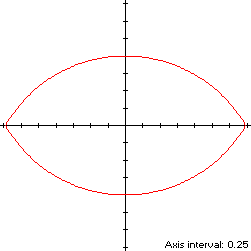
The Vesica Piscis (red) is a member of a group of similarly-shaped figures described on these pages, including (inside to outside) the mouth curve and the cycloid:
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.