Tschirnhausen Cubic

Tschirnhausen Cubic TSCHIRNHAUSEN CUBIC

Balmoral Software

The lobe of the Tschirnhausen cubic is a convex x-symmetric droplet-shaped closed curve S with polar equation

r(t) = sec³(t/3), -π ≤ t < π

The curve follows a counterclockwise path as t increases, starting from the cusp (-8,0) at t = -π and proceeding through Quadrants III and IV to the right edge (1,0) at t = 0, then following a symmetric path through Quadrants I and II back to the cusp at t = π. The maximum ordinate of the Tschirnhausen cubic occurs at (-2,2) when t = 3π/4, so the width x height of its bounding rectangle is 9 x 4.

Metrics

We have

r'(t) = tan(t/3)sec³(t/3),

so by (L2), the perimeter of the Tschirnhausen cubic is

and by (A2), its area A is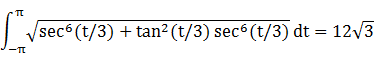

By (C2), its centroid abscissa is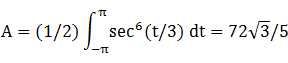

Incircle

The maximum radius of a circle centered on the x-axis and inscribed in S is its maximum ordinate, so a candidate for the incircle has radius R = 2 and center abscissa c = -2. The candidate circle must be contained within S, so we require that c - R and c + R both be within the abscissa range [-8,1] of S, which is true. For verification, we have

Inellipse

Using z = 1 in Lemma E,

d/dt [x(t) - z]y(t) = d/dt -3sin(t)tan²(t/3)sec³(t/3)

has a zero at t* = (3/2)Arccos(-2/7). The corresponding coordinates are

We then have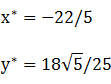

For verification,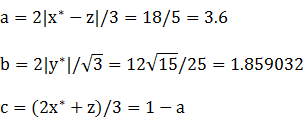

Circumellipse

Using z = -8 in Lemma E,

d/dt [x(t) - z]y(t) = d/dt [sec³(t/3)cos(t) + 8]sec³(t/3)sin(t)

has a zero at

The corresponding coordinates are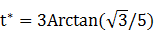

We then have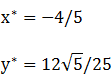

For verification,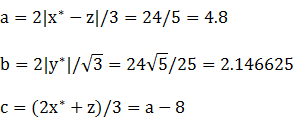

Circumcircle

Since the abscissa extrema are on the x-axis and the bounding rectangle is wider than it is tall, a candidate for the circumcircle has radius 9/2 and center abscissa -7/2. For verification, we have

Summary Table

Figure Parameters Perimeter Area Centroid

Incircle R = 2 12.566371 12.566371 (-2,0)

Inellipse 17.588970 21.025157 (-2.6,0)

Tschirnhausen cubic Width: 9
Height: 4 20.784610 24.941532 (-2.857143,0)

Circumellipse 22.627006 32.370342 (-3.2,0)

Circumcircle R = 9/2 28.274334 63.617251 (-3.5,0)

Figure	Parameters	Perimeter	Area	Centroid
Incircle	R = 2	12.566371	12.566371	(-2,0)
Inellipse		17.588970	21.025157	(-2.6,0)
Tschirnhausen cubic	Width: 9 Height: 4	20.784610	24.941532	(-2.857143,0)
Circumellipse		22.627006	32.370342	(-3.2,0)
Circumcircle	R = 9/2	28.274334	63.617251	(-3.5,0)

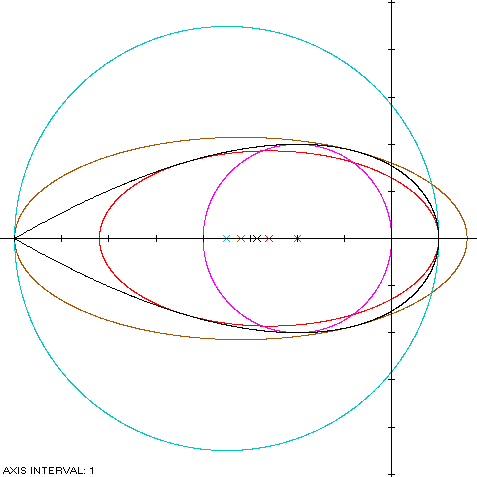
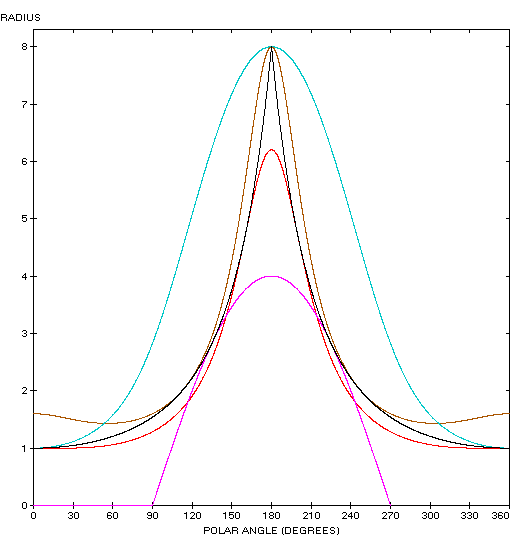
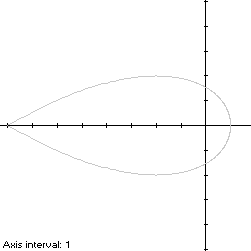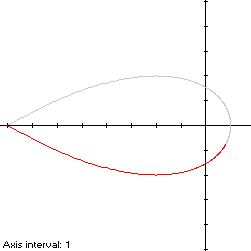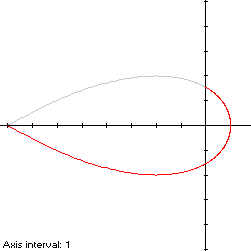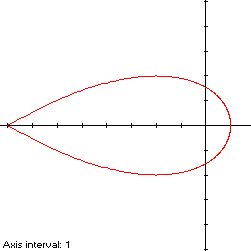
The Tschirnhausen cubic (red) is a member of a group of similarly-shaped figures described on these pages, including (inside to outside) the teardrop curve, the right strophoid, the Trisectrix of Maclaurin and the piriform curve:

Top Page

Home