Trisectrix of Maclaurin

Trisectrix of Maclaurin TRISECTRIX OF MACLAURIN

Balmoral Software

The lobe of the Trisectrix of Maclaurin is a convex x-symmetric droplet-shaped closed curve S with polar equation

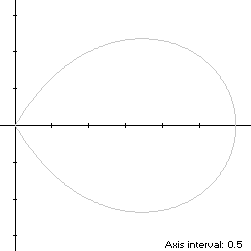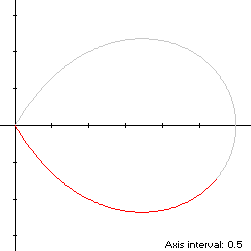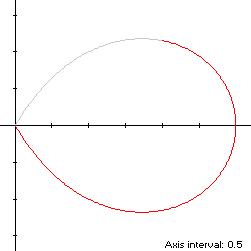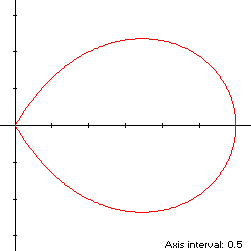
r(t) = 4 cos(t) - sec(t), -π/3 ≤ t < π/3

The curve follows a counterclockwise path as t increases, starting from the cusp at the origin when t = -π/3, proceeding through Quadrant IV to the right edge (3,0) at t = 0, then following a symmetric path through Quadrant I back to the cusp at t = π/3. The maximum ordinate point of S is

so the width x height of its bounding rectangle is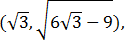

Metrics

The area of S is

and its perimeter is 8.244653. By (C2), its centroid abscissa is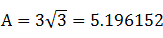

Incircle

The maximum radius of a circle centered on the x-axis and inscribed in S is its maximum ordinate, so a candidate for the incircle has radius

and center abscissa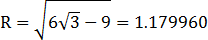

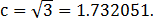The candidate circle must be contained within S, so we require that c - R and c + R both be within the abscissa range [0,3] of S, which is true. For verification, we have

Inellipse

Using z = 3 in Lemma E,

d/dt [x(t) - z]y(t) = d/dt -4sin²(t)tan(t)[2cos(2t) + 1]

has a zero at t* = π/4. The corresponding coordinates are (x*,y*) = (1,1). We then have

For verification,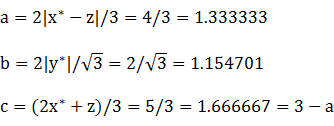

Circumellipse

Using z = 0 in Lemma E,

d/dt [x(t) - z]y(t) = d/dt [4cos(t) - sec(t)]²cos(t)sin(t)

has a zero at

The corresponding coordinates are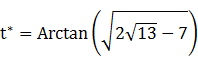

We then have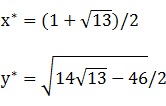

For verification,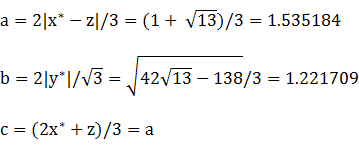

Circumcircle

Since the abscissa extrema are on the x-axis and the bounding rectangle is wider than it is tall, a candidate for the circumcircle has its radius and center abscissa both equal to 3/2. For verification, we have

Summary Table

Figure Parameters Perimeter Area Centroid

Incircle R = 7.413905 4.374055 (1.732051,0)

Inellipse 7.826465 4.836799 (1.666667,0)

Trisectrix 8.244653 5.196152 (1.612266,0)

Circumellipse 8.689052 5.892208 (1.535184,0)

Circumcircle R = 3/2 9.424778 7.068584 (1.5,0)

Figure	Parameters	Perimeter	Area	Centroid
Incircle	R =	7.413905	4.374055	(1.732051,0)
Inellipse		7.826465	4.836799	(1.666667,0)
Trisectrix		8.244653	5.196152	(1.612266,0)
Circumellipse		8.689052	5.892208	(1.535184,0)
Circumcircle	R = 3/2	9.424778	7.068584	(1.5,0)

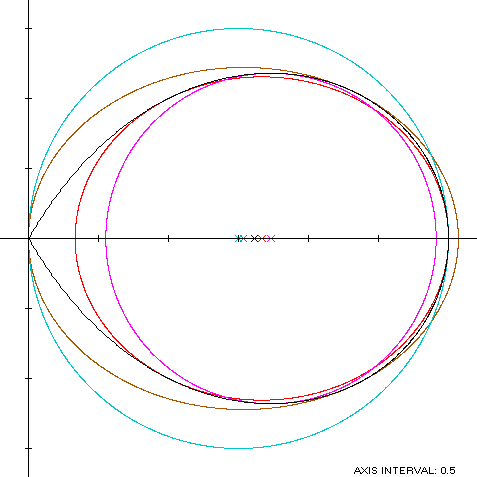
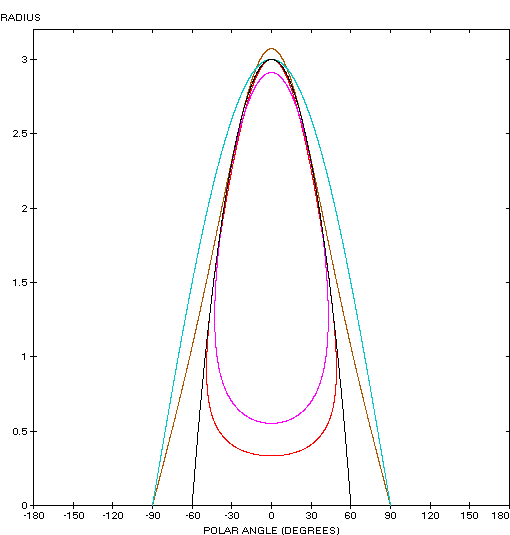
The Trisectrix of Maclaurin (red) is a member of a group of similarly-shaped figures described on these pages, including (inside to outside) the teardrop curve, the Tschirnhausen cubic, the right strophoid and the piriform curve:

Relationship with the Folium of Descartes

The Folium of Descartes coincides with the Trisectrix of Maclaurin after a rotation and non-uniform scaling of the coordinates. The polar equation of the Folium

is rotated clockwise by π/4, producing the new x-symmetric polar function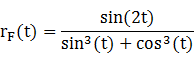

Over the lobe domain -π/4 ≤ t < π/4, the maximum abscissa and ordinate of the rotated Folium are

so to scale the Folium to the comparable coordinate extrema of the trisectrix, we multiply its abscissas by 3/x_max and its ordinates by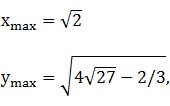

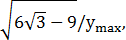producing the coordinate functions

These coordinate functions cannot be directly compared with the corresponding ones for the trisectrix since the rates of travel along the path (if t is considered a time variable) vary between the two curves, and the domains for t are different. However, the path itself is the same for both curves since the preceding x(t), y(t) satisfy the cartesian form of the trisectrix: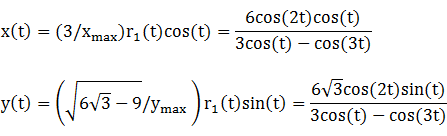

2x(x² + y²) = 2(3x² - y²)

Top Page

Home