Balmoral Software
Balmoral Software

Let t represent the usual parameter, ranging from 0 to 2π through the quadrants in counterclockwise order. In Quadrants I and II, the parametric equations of the segment arc are:
x(φ) = cos(φ)where the angle φ is measured from the center of the circle and ranges fromy(φ) = sin(φ) - cos(β),
or equivalently,
Range of t Range of φ [0,π] [π/2 - β,π/2 + β]
φ = 2βt/π + π/2 - βIn Quadrants III and IV, the parametric function x(t) of the horizontal chord is linear in t. Combining definitions, we have
L = 2β + 2sin(β)The area of S is
A = [2β - sin(2β)]/2and its centroid ordinate is
Specific Example
In the remainder of this paper, we use
β = 35° = 7π/36L = 2.368883
A = 0.141019
yc = 0.072931
Bounding rectangle: 1.147153 x 0.180848
R = d = [1 - cos(β)]/2 = 0.090424For verification, we have
d/dt x(t)[y(t) - z] = d/dt cos(2βt/π + π/2 - β)[sin(2βt/π + π/2 - β) - cos(β)]has a zero over [0,π) at t* = 0.682719. The corresponding coordinates are
x* = 0.338539We then havey* = 0.121800
For verification,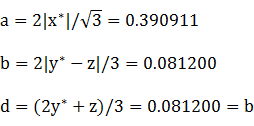
For verification,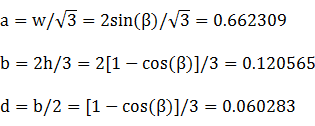
R = sin(β) = 0.573576Since the radius of the circumcircle is less than the unit radius of the segment, the circumcircle has greater curvature and thus encloses S.d = 0
Figure Parameters Perimeter Area Centroid Incircle R = [1 - cos(β)]/2 0.568151 0.025687 (0,0.090424) Inellipse a = 0.390911
b = 0.0812001.649400 0.099720 (0,0.081200) Circular segment Width: 2sin(β)
Height: 1 - cos(β)2.368883 0.141019 (0,0.072931) Circumellipse a =
b =2.765929 0.250860 (0,0.060283) Circumcircle R = sin(β) 3.603887 1.033552
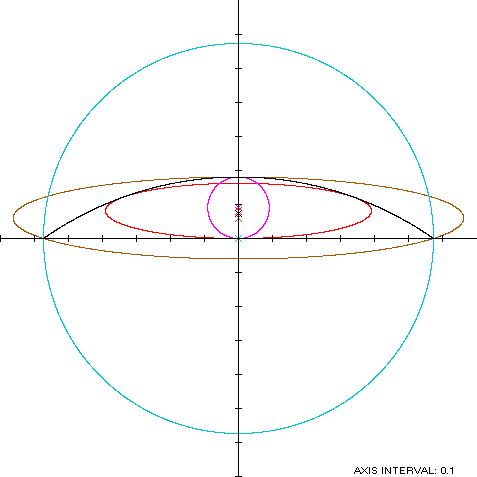
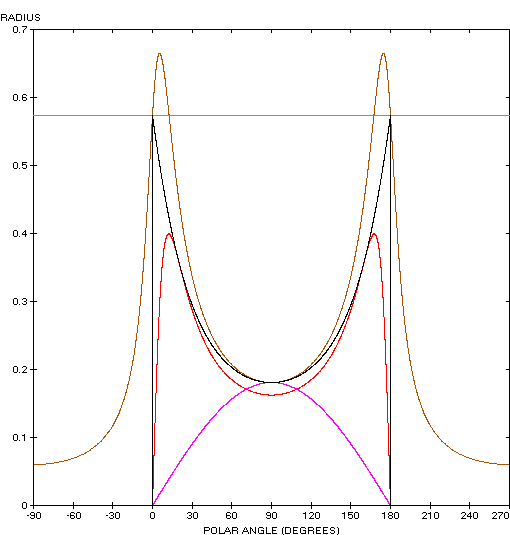
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.