Balmoral Software
Balmoral Software

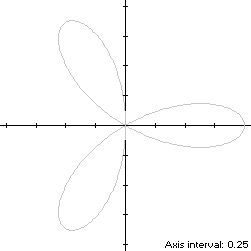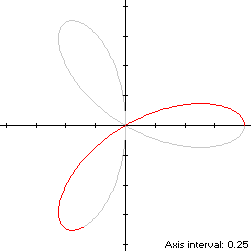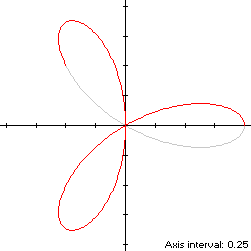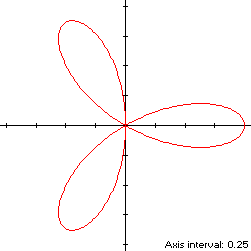
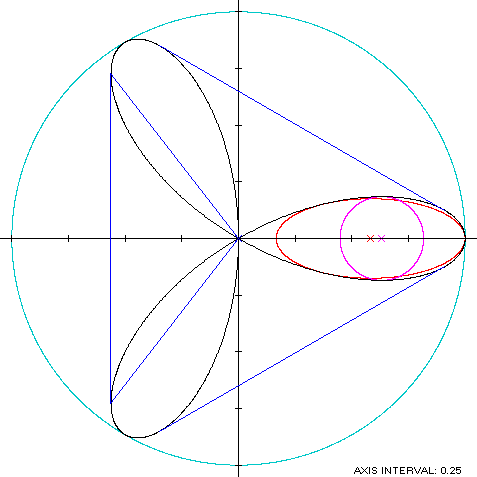
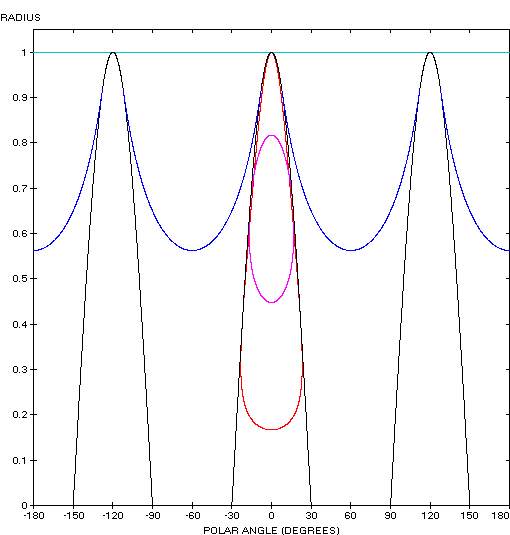
r(t) = cos(3t), 0 ≤ t < πThis curve is also known as the trifolium. Movement along S is always counterclockwise, starting at its maximum abscissa point (1,0) and crossing the origin three times. There are two minimum-abscissa points
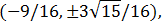 the lower of which occurs at
the lower of which occurs at
so S is non-convex by the multiple local extrema test. The ordinate extrema of S are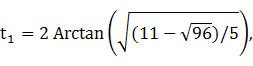
so the width x height of its bounding rectangle is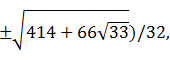
r(t + 2π/3) = cos(3t + 2π) = r(t),the condition (R2) of Lemma R is satisfied and the rose is rotationally symmetric with period 2π/3.
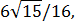 and then repeating the pattern by rotating around the origin at 2π/3
intervals. The far end of the lower-left rose petal is reached at
and then repeating the pattern by rotating around the origin at 2π/3
intervals. The far end of the lower-left rose petal is reached at
r'(t) = -3sin(3t),so by (L2), the perimeter of the convex hull is
which is about 20% shorter than that of the rose.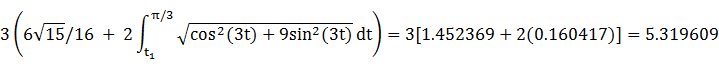
The line segments of the convex hull create three isosceles triangles with the origin, each having an area of
as shown in blue in the left diagram below. By (A2), the area of the convex hull is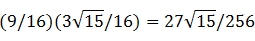
which is a little over twice the area of the rose.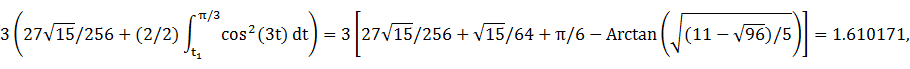
r2(t) = cos2(3t)is maximized at 1, so that is the circumradius.
and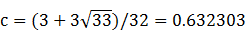
The largest circle centered on the x-axis and inscribed in the lobe has radius R and center abscissa c. The circle must be contained within the lobe, so we require that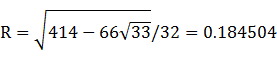
d/dt [x(t) - z]y(t) = d/dt [6sin(2t) - 5sin(4t) + sin(8t)]/8has a zero at
The corresponding coordinates are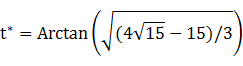
We then have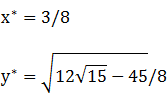
For verification, we have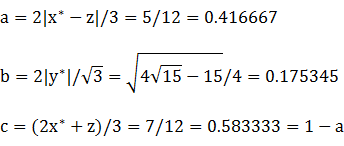
Figure Parameters Perimeter Area Centroid Incircle (lobe) R = 1.159273 0.106945 (0.632303,0) Inellipse (lobe) a = 0.416666
b = 0.1753451.937955 0.229526 (0.583334,0) Rose 6.682447 0.785398 Convex hull 5.319609 1.610171 Circumcircle R = 1 6.283185 3.141593
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.