Lima Bean Curve

Lima Bean Curve LIMA BEAN CURVE

Balmoral Software

The lima bean curve is a tilted closed curve with polar equation

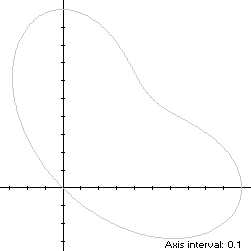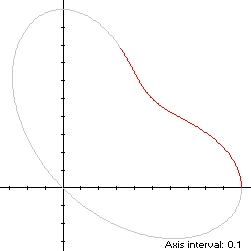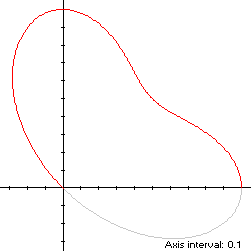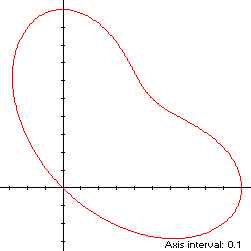
r(t) = sin³(t) + cos³(t), 0 ≤ t < π

The curve is traced around and through the origin in a counterclockwise direction, starting from its maximum abscissa point (1,0) at t = 0, reaching its closest approach to the origin in the first quadrant at (1/2,1/2) when t = π/4, then its maximum ordinate point (0,1) at t = π/2 and through Quadrant II to the origin at t = 3π/4 before returning through Quadrant IV to its starting point. Its minimum abscissa and ordinate points are (-0.282887,0.621170) and (0.621170,-0.282887), respectively, so its bounding rectangle is a 1.282887 x 1.282887 square.

Metrics

The perimeter of the lima bean curve is 3.931703 and its area is 5π/16. Its centroid is the point (3/10,3/10).

Rotated Curve

Since

r(π/4 + t) = r(π/4 - t),

the lima bean curve is symmetric with respect to the line y = x, and is more amenable to analysis after being rotated clockwise by π/4, producing an x-symmetric curve S:

The polar function of the rotated curve is: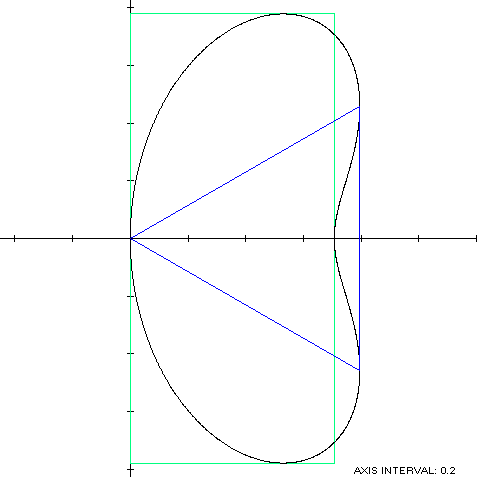

r_S(t) = sin³(t + π/4) + cos³(t + π/4), 0 ≤ t < π,

and the corresponding coordinate functions are

x(t) = r_S(t)cos(t) = [sin³(t + π/4) + cos³(t + π/4)]cos(t)
y(t) = r_S(t)sin(t) = [sin³(t + π/4) + cos³(t + π/4)]sin(t)

The minimum abscissa point of S is the origin at t = π/2; and its two maximum abscissa points are

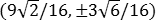at t = π/6 and t = 5π/6, so S and the lima bean curve are non-convex by the multiple local extrema test. The maximum ordinate point of S is

The bounding rectangle for inconics is delimited by the ordinate extrema and the cusp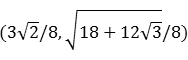

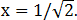
Convex Hull

The convex hull is created by connecting the maximum abscissa points with a vertical line segment of length

We have

so by (L2), the perimeter of the convex hull is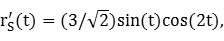

which is less than 1% shorter than that of the lima bean curve.

The line segment of the convex hull creates an isosceles triangle with the origin, having area

as shown in blue in the diagram above. The centroid abscissa of this triangle is the average of its vertex abscissas, or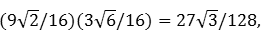

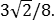By (A2), the remainder of the convex hull has area

By (C2), the associated centroid abscissa is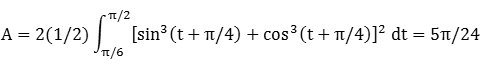

The convex hull component metrics can be summarized as follows: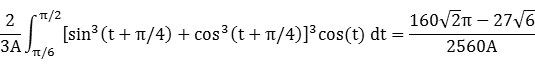

Region Area Centroid abscissa Product

Triangle

Remainder

Total

The area of the convex hull is 1.019853, about 4% larger than that of the lima bean curve.

The centroid abscissa of the convex hull of S is the weighted average

After a counterclockwise rotation by π/4, the centroid's position with respect to the lima bean curve is (0.308956,0.308956).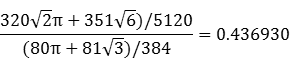

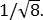Incircle

A candidate incircle is located on the x-axis between the origin and the cusp, and so has radius R and center abscissa c both equal to

For verification, we have

After a counterclockwise rotation by π/4, the incircle's center is (1/4,1/4).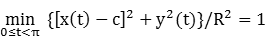

Inellipse

Using

in Lemma E,

d/dt [x(t) - z]y(t) = d/dt (-1/8)sin³(t)[-2cos(t) - 3cos(3t) + cos(5t)]

has a zero at t* = 1.245619. The corresponding coordinates are

x* = 0.201781
y* = 0.598499

We then have

For verification,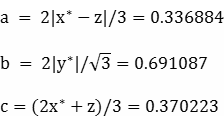

Circumellipse

Using z = 0 in Lemma E,

d/dt [x(t) - z]y(t) = d/dt [26sin(2t) + 2sin(4t) - 6sin(6t) + sin(8t)]/64

has a zero at t* = π/4. The corresponding coordinates are

We then have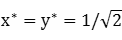

For verification,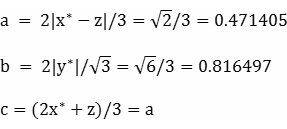

Circumcircle

The radius of a circle centered on the x-axis and circumscribing S is at least its maximum ordinate, so a candidate for the circumcircle has radius

and center abscissa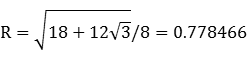

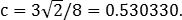For verification,

After a counterclockwise rotation by π/4, the circumcircle's center is (3/8,3/8).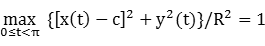

Summary Table

Figure Parameters Perimeter Area Centroid

Incircle 2.221442 0.392699 (0.25,0.25)

Inellipse * a = 0.336884
b = 0.691087 3.326054 0.731414 (0.370223,0)

Lima bean curve Width: 1.282887
Height: 1.282887 3.931703 0.981748 (0.3,0.3)

Convex hull 3.911279 1.019853 (0.308956,0.308956)

Circumellipse * 4.119019 1.209201 (0.471405,0)

Circumcircle 4.891247 1.903835 (0.375,0.375)

*: With respect to rotated curve S

Figure	Parameters	Perimeter	Area	Centroid
Incircle		2.221442	0.392699	(0.25,0.25)
Inellipse *	a = 0.336884 b = 0.691087	3.326054	0.731414	(0.370223,0)
Lima bean curve	Width: 1.282887 Height: 1.282887	3.931703	0.981748	(0.3,0.3)
Convex hull		3.911279	1.019853	(0.308956,0.308956)
Circumellipse *		4.119019	1.209201	(0.471405,0)
Circumcircle		4.891247	1.903835	(0.375,0.375)

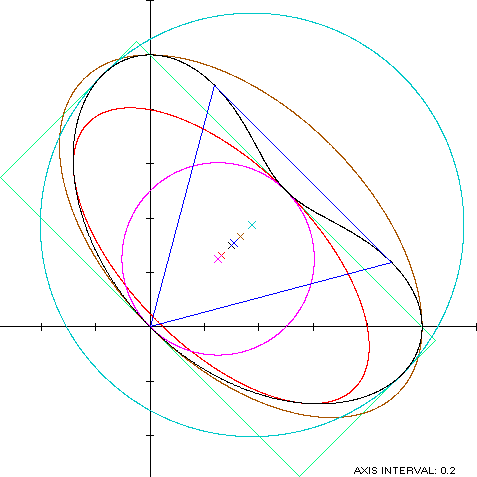
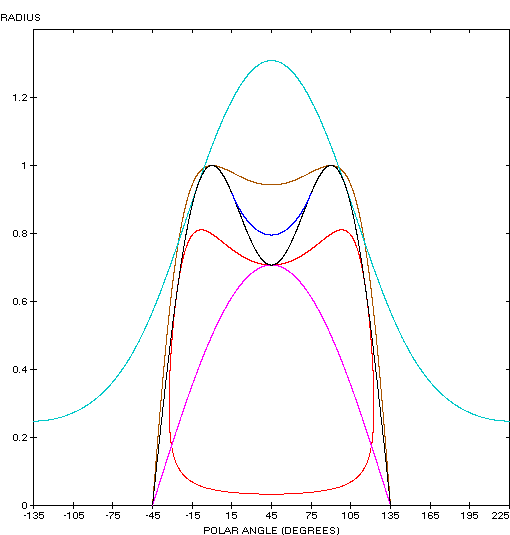
The rotated lima bean curve (red) is a member of a group of similarly-shaped figures described on these pages, including (inside to outside) the cardioid, the cochleoid and the Cayley sextic.

Top Page

Home

Region	Area	Centroid abscissa	Product
Triangle
Remainder
Total