Heart Curve

Heart Curve HEART CURVE

Balmoral Software

The fifth heart curve is an algebraic eighth-degree y-symmetric closed curve S defined by the parametric equations

x(t) = 16sin³(t)
y(t) = 13cos(t) - 5cos(2t) - 2cos(3t) - cos(4t)

for t ∈ T = [0,2π). The curve follows a clockwise path as t increases, starting from the cusp (0,5) at t = 0, reaching its maximum-abscissa point (16,4) at t = π/2, its minimum-ordinate point (0,-17) at t = π, and then following a symmetric path back to the cusp. Since maximum-ordinate points (±7.838207,11.923252) occur at

t₁ = 0.908063

and at -t₁ in the two bulges of the heart, S is non-convex by the multiple local extrema test, and the width x height of its bounding rectangle is 32 x 28.923252.

Metrics

We have

x'(t) = 48sin²(t)cos(t)
y'(t) = -13sin(t) + 10sin(2t) + 6sin(3t) + 4sin(4t),

so by (L1), the perimeter of the heart curve is

and its area is A = 180π. By (C1), its centroid ordinate is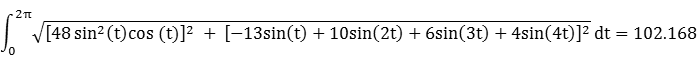

The bounding rectangle for inconics is delimited by the cusp at (0,5).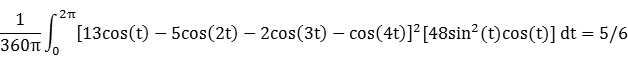

Convex Hull

The top part of the convex hull of the heart curve is created by connecting the two maximum-ordinate points with a line segment of length 2x(t₁) = 15.676414. We have

x''(t) = -12sin(t) + 36sin(3t)
y''(t) = -13cos(t) + 20cos(2t) + 18cos(3t) + 16cos(4t),

so by (X1), the curvature of S changes sign at (0,-17) since

48sin²(t)cos(t)[-13cos(t) + 20cos(2t) + 18cos(3t) + 16cos(4t)] = [-12sin(t) + 36sin(3t)][-13sin(t) + 10sin(2t) + 6sin(3t) + 4sin(4t)]

is satisfied for t = π. Equation (X2) is evaluated with respect to the point (0,-17) as

which has a solution in the right half-plane at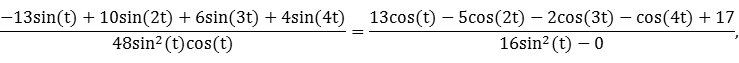

t₂ = 1.973512,

so convex hull line segments extend between the points (0,-17) and (±x(t₂),y(t₂)) = (±12.459008,-3.460803). These line segments are shown in dark blue at the bottom of the left diagram below, and each has length 18.399368. By (L1), the perimeter of the convex hull is:

which is about 7% shorter than that of the heart curve.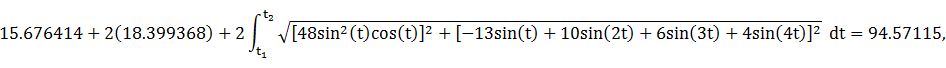

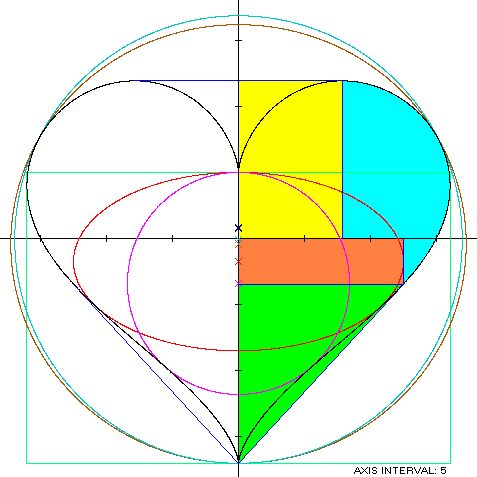
The right half of the convex hull can be partitioned into four areas, shown in color in the left diagram below:

A rectangle P₁ (yellow) with corners at the origin and at the maximum-ordinate point (x(t₁),y(t₁)).
A right trapezoid containing all the Quadrant IV space between the lower-right convex hull line segment and the vertical line at x(t₂). For convenience, the trapezoid is separated into a rectangle P₂ (orange) with corners at the origin and at (x(t₂),y(t₂)), and a triangle P₃ (green) containing the remainder of the trapezoid.
A comma-shaped region P₄ (light blue) delimited by the curve S between t₁ and t₂ and the boundaries of P₁ and P₂.

The centroid ordinate of each rectangle and triangle is the average of its vertex ordinates. By (A1), the area of the comma-shaped region P₄ is

and by (C1), its centroid ordinate is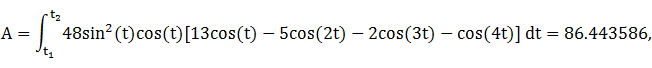

The half-hearted convex hull component metrics can be summarized as follows: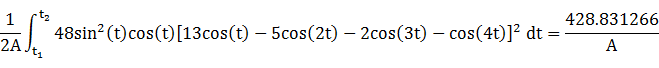

Region Area Centroid ordinate Product

P₁ x(t₁)y(t₁) = 93.456921 y(t₁)/2 x(t₁)y²(t₁)/2 = 557.155231

P₂ ∣x(t₂)y(t₂)∣ = 43.118171 y(t₂)/2 -x(t₂)y²(t₂)/2 = -74.611741

P₃ x(t₂)[y(t₂) + 17]/2 = 84.342486 [2y(t₂) - 17]/3 x(t₂)[y(t₂) + 17][2y(t₂) - 17]/6 = -672.535896

P₄ A = 86.443586 428.831266/A 428.831266

Total 307.361164 238.838860

Region	Area	Centroid ordinate	Product
P₁	x(t₁)y(t₁) = 93.456921	y(t₁)/2	x(t₁)y²(t₁)/2 = 557.155231
P₂	∣x(t₂)y(t₂)∣ = 43.118171	y(t₂)/2	-x(t₂)y²(t₂)/2 = -74.611741
P₃	x(t₂)[y(t₂) + 17]/2 = 84.342486	[2y(t₂) - 17]/3	x(t₂)[y(t₂) + 17][2y(t₂) - 17]/6 = -672.535896
P₄	A = 86.443586	428.831266/A	428.831266
Total	307.361164		238.838860

The area of the entire convex hull then is 614.722328, about 9% larger than the area of the heart curve. The centroid ordinate of the convex hull is the weighted average

Incircle

Using z = 5 in Lemma C, we have

and R = |d - z| = 8.39809. For verification, we have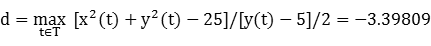

Inellipse

Using z = 5 in Lemma E,

has a zero at t* = 2.071360. The corresponding coordinates are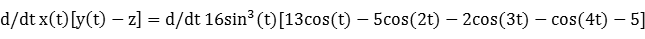

x* = 10.8039
y* = -5.11923

We then have

For verification,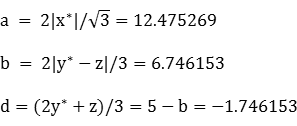

Circumellipse

Using z = -17 in Lemma E,

has a zero at t* = 1.355197. The corresponding coordinates are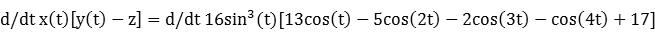

x* = 14.9143
y* = 7.8781

We then have

For verification,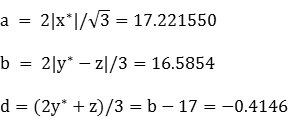

Circumcircle

Using z = -17 in Lemma C,

and R = |d - z| = 16.922074. For verification, we have

The optimal circumcircle is slightly smaller than an origin-centered circle with radius = 17 exactly.

Summary Table

Figure Parameters Perimeter Area Centroid

Incircle R = 8.39809 52.766756 221.569982 (0,-3.39809)

Inellipse a = 12.475269
b = 6.746153 61.734653 264.396668 (0,-1.746153)

Heart curve Width: 32
Height: 28.923252 102.168 565.486678 (0,0.833333)

Convex hull 94.57115 614.722328 (0,0.777063)

Circumellipse a = 17.221550
b = 16.585373 106.216983 897.320010 (0,-0.414627)

Circumcircle R = 16.922074 106.324527 899.615755 (0,-0.077926)

Figure	Parameters	Perimeter	Area	Centroid
Incircle	R = 8.39809	52.766756	221.569982	(0,-3.39809)
Inellipse	a = 12.475269 b = 6.746153	61.734653	264.396668	(0,-1.746153)
Heart curve	Width: 32 Height: 28.923252	102.168	565.486678	(0,0.833333)
Convex hull		94.57115	614.722328	(0,0.777063)
Circumellipse	a = 17.221550 b = 16.585373	106.216983	897.320010	(0,-0.414627)
Circumcircle	R = 16.922074	106.324527	899.615755	(0,-0.077926)

Top Page

Home