Lemniscate of Gerono

Lemniscate of Gerono LEMNISCATE OF GERONO

Balmoral Software

The Lemniscate of Gerono is a bisymmetric figure-eight curve S with parametric equations

x(t) = sin(t)
y(t) = sin(2t)/2, 0 ≤ t < 2π

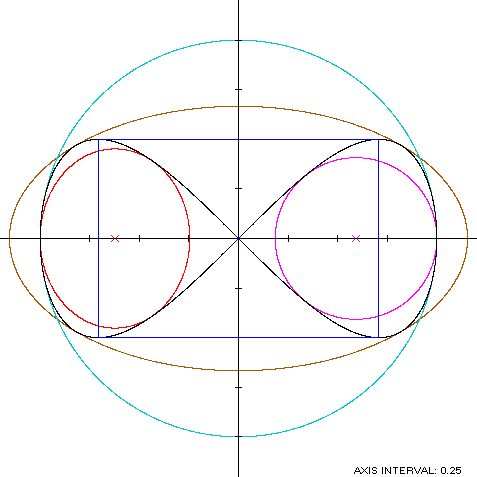
The path of S starts at the origin, passes clockwise through Quadrants I and IV, then counterclockwise through Quadrants II and III and back to the origin. Its abscissa extrema are on the x-axis at ±1 when 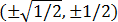t = ±π/2, and its extreme ordinate points are

at t = ±π/4, ±3π/4, so the width x height of its bounding rectangle is 2 x 1.

Metrics

The perimeter of S is 6.097223 (OEIS A118178) and its area is 4/3.

Convex Hull

The convex hull is created by connecting the extreme ordinate points with two horizontal line segments of length

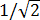. We have

x'(t) = cos(t)
y'(t) = cos(2t),

so by (L1), the perimeter of the convex hull is

which is about 12% shorter than that of the lemniscate.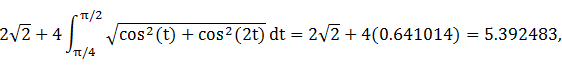

The line segments of the convex hull create a rectangle of area 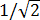, as shown in blue in the left diagram below. By (A1), the area of the convex hull is

which is about 41% more than the area of the lemniscate.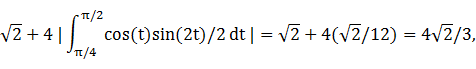

Circumcircle

The maximum squared distance

x²(t) + y²(t) = sin²(t) + sin²(2t)/4

is 1, so that is the circumradius.

Circumellipse

From Lemma B, we have

x(t)y(t) = sin(t)sin(2t)/2

This expression is maximized in the first quadrant at

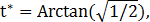so the circumellipse dimensions are

For verification, we have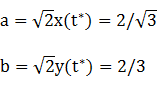

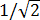Incircle (lobe)

Consider the right lobe of the lemniscate, where 0 ≤ t < π. The maximum ordinate 1/2 of the lobe does not define its inradius since the corresponding abscissa

is too close to the right edge (1,0), so its incircle is constrained by the right edge of S. Using z = 1 in Lemma C, we have

and R = |c - z| = 11/27. For verification, we have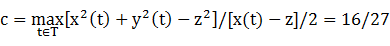

Inellipse (lobe)

Using z = 1 in Lemma E,

d/dt [x(t) - z]y(t) = [sin(t) - 1]sin(2t)/2

has a zero at

The corresponding coordinates are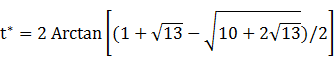

We then have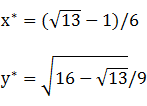

For verification, we have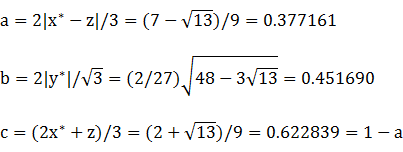

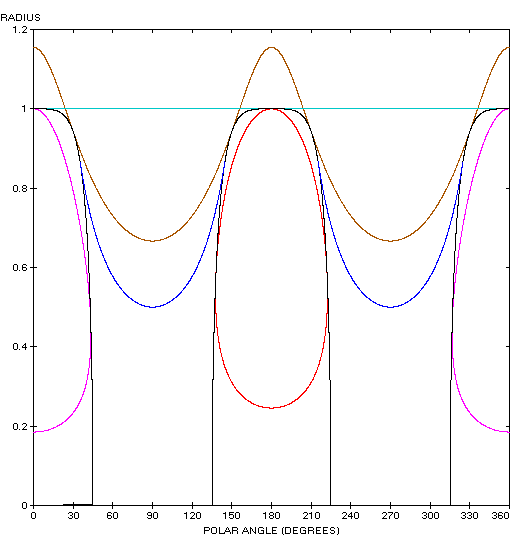
Summary Table

Figure Parameters Perimeter Area Centroid

Incircle (lobe) R = 11/27 2.559816 0.521444 (0.592593,0)

Inellipse (lobe) 2.609178 0.535201 (0.622839,0)

Lemniscate of Gerono Width: 2
Height: 1 6.097223 1.333333

Convex hull 5.392483 1.885618

Circumellipse 5.825171 2.418401

Circumcircle R = 1 6.283185 3.141593

Figure	Parameters	Perimeter	Area	Centroid
Incircle (lobe)	R = 11/27	2.559816	0.521444	(0.592593,0)
Inellipse (lobe)		2.609178	0.535201	(0.622839,0)
Lemniscate of Gerono	Width: 2 Height: 1	6.097223	1.333333
Convex hull		5.392483	1.885618
Circumellipse		5.825171	2.418401
Circumcircle	R = 1	6.283185	3.141593


Binoculars

The Lemniscate of Gerono (red) is a member of a group of figure-8 curves described on these pages, including (inside to outside) the dumbbell curve, the bowtie, the Lemniscate of Bernoulli and the dipole:

Top Page

Home