Balmoral Software
Balmoral Software

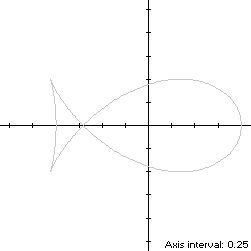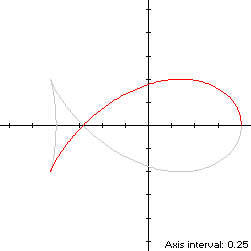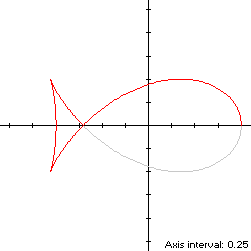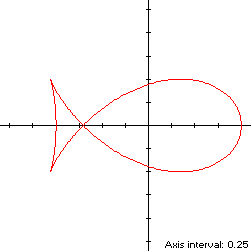
for t ∈ T = [0,2π). As t increases from 0, S starts from its right-hand edge at (1,0) and moves through Quadrants I and II, crossing the x-axis at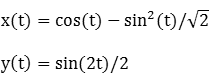
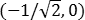 when
when 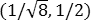 when
when 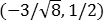 when
when
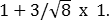
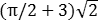 and its area is 4/3. Its
centroid
is
and its area is 4/3. Its
centroid
is 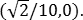
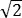 and one vertical line segment
of length 1. We have
and one vertical line segment
of length 1. We have
so by (L1) and symmetry, the perimeter of the convex hull is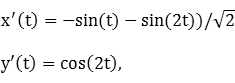
which is about 13% shorter than that of the fish curve.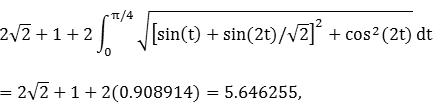
The line segments of the convex hull create a rectangle of area
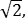 as shown in blue in the left
diagram below. The centroid abscissa of this rectangle is the average of its
vertex abscissas, or
as shown in blue in the left
diagram below. The centroid abscissa of this rectangle is the average of its
vertex abscissas, or 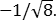 By
(A1) and symmetry, the remainder of the
convex hull has area
By
(A1) and symmetry, the remainder of the
convex hull has area
By (C1), the associated centroid abscissa is found by integrating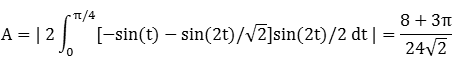
Since S is defined parametrically and is traced out in a counterclockwise direction, the usual limits of integration are reversed.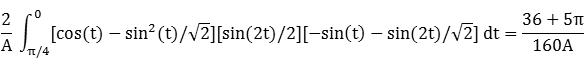
The convex hull component metrics can be summarized as follows:
The area of the convex hull is 1.927596, about 45% larger than that of the fish curve. The centroid abscissa of the convex hull is the weighted average
Region Area Centroid abscissa Product Rectangle -1/2 Remainder Total
and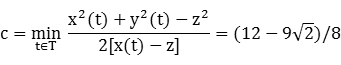
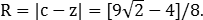 For verification, we have
For verification, we have
a = 1.290478b = 0.640445
c = -0.254244
d = 0
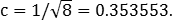 The candidate circle must be
contained within S, so we require that
The candidate circle must be
contained within S, so we require that 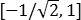 of the main lobe of S, which is
true. For verification, we have
of the main lobe of S, which is
true. For verification, we have
has a zero when t* is a complicated expression approximated by 1.083243 radians. The corresponding coordinates are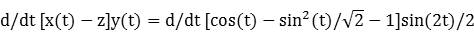
x* = -0.083459from which we havey* = 0.413881,
For verification,
has a zero when t* is a complicated expression approximated by 1.749441 radians. The corresponding coordinates are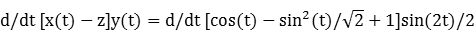
x* = -0.862475from which we havey* = -0.174868,
For verification, we have
Figure Parameters Perimeter Area Centroid Incircle (tail) 0.774771 0.047768 (-0.876691,0) Inellipse (tail) a = 0.091683
b = 0.2019200.955186 0.058159 (-0.908317,0) Incircle (main lobe) R = 1/2 3.141593 0.785398 (0.353553,0) Inellipse (main lobe) a = 0.722306
b = 0.4779083.809772 1.084465 (0.277694,0) Fish curve 6.464082 1.333333 (0.141421,0) Convex hull 5.646255 1.927596 (-0.129731,0) Circumellipse a = 1.290478
b = 0.6404456.239295 2.596464 (-0.254244,0) Circumcircle 6.854894 3.739311 (-0.090990,0)
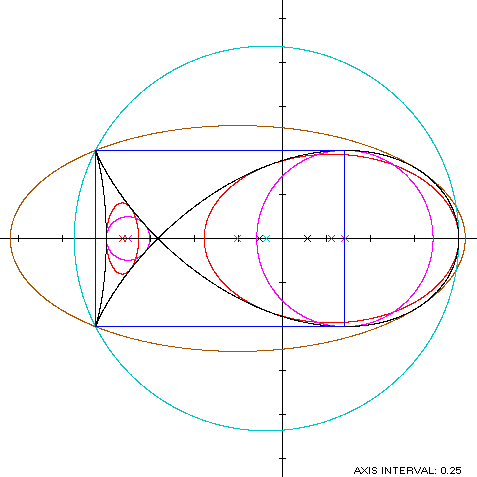
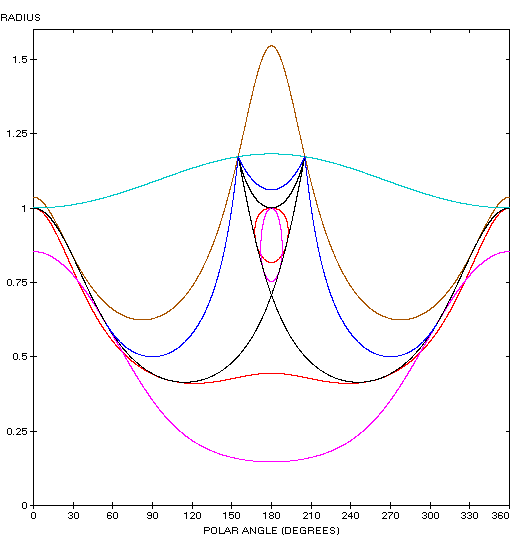
The fish curve (red) is similarly shaped to the trefoil:
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.