Balmoral Software
Balmoral Software

p(t) = 2 sin(2t) + 1, 0 ≤ t < 2πRotating the curve clockwise by π/4 produces the equivalent bisymmetric curve S with polar equation
r(t) = p(t + π/4) = 2 cos(2t) + 1, 0 ≤ t < 2πThe corresponding implicit function can be found by replacing cos(t) with x/r and r with
(x2 + y2)3 = (3x2 - y2)2The corresponding parametric functions are:
x(t) = 2cos(t) + cos(3t)Movement along S is always counterclockwise, crossing the origin four times. Aty(t) = sin(3t)
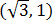 at
at 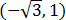 at
at
Since S has three maximum-ordinate points, it is non-convex by the multiple local extrema test. The width x height of the bowtie's bounding rectangle is 6 x 2.
t: 0 π/6 π/3 π/2 2π/3 5π/6 π 7π/6 4π/3 3π/2 5π/3 11π/6 2π x(t): 3 0 0 0 - -3 - 0 0 0 3 y(t): 0 1 0 -1 0 1 0 -1 0 1 0 -1 0
r'(t) = -4 sin(2t),so by (L2), the perimeter of S is
and by (A2), the area of S is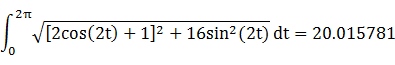
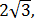 each of which is bisected by
another extreme ordinate point on one of the small lobes. By
(L2), the perimeter of the convex hull is
each of which is bisected by
another extreme ordinate point on one of the small lobes. By
(L2), the perimeter of the convex hull is
which is about 30% shorter than that of the bowtie.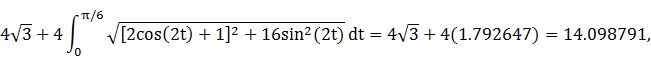
The line segments of the convex hull create two isosceles triangles with the origin, each having an area of
as shown in blue in the left diagram below. By (A2), the area of the convex hull is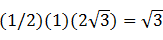
which is about 16% more than the area of the bowtie.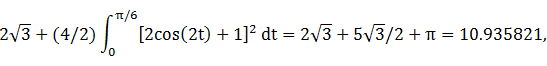
x(t)y(t) = [2cos(t) + cos(3t)]sin(3t)This expression is maximized in the first quadrant at the complicated value
The circumellipse dimensions are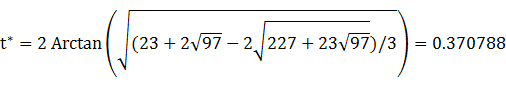
For verification, we have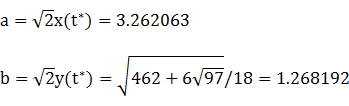
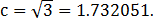 The circle must be contained
within the lobe, so we require that
The circle must be contained
within the lobe, so we require that d/dt [x(t) - z]y(t) = 2 cos(2t) - 9 cos(3t) + 4 cos(4t) + 3 cos(6t)has a zero at t* = 0.711832. The corresponding coordinates are
x* = 0.979171We then havey* = 0.844749
For verification, we have
Figure Parameters Perimeter Area Centroid Incircle (lobe) R = 1 6.283185 3.141593 (1.732051,0) Inellipse (lobe) a = 1.347219
b = 0.9754327.343639 4.128431 (-1.652781,0) Bowtie Width: 6
Height: 220.015781 9.424778 Convex hull 14.098791 10.935821 Circumellipse a = 3.262063
b = 1.26819214.930224 12.996524 Circumcircle R = 3 18.849556 28.274334
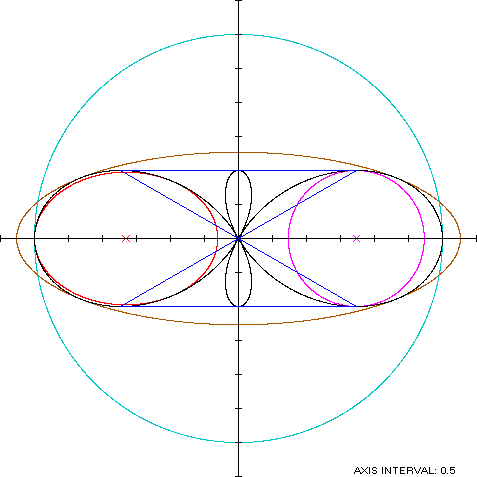
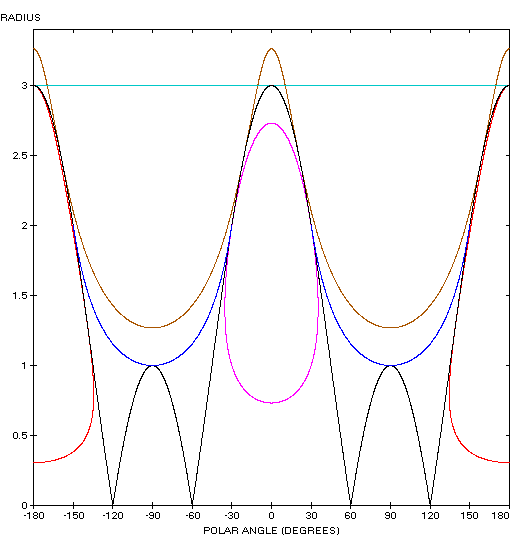
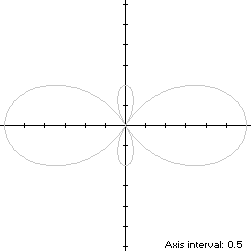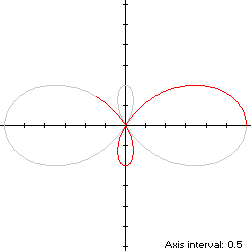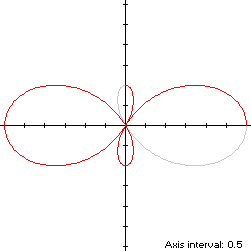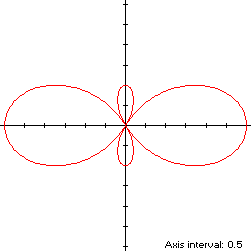
The bowtie curve (red) is a member of a group of figure-8 curves described on these pages, including (inside to outside) the dumbbell curve, the Lemniscate of Bernoulli, the Lemniscate of Gerono and the dipole:
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.