Balmoral Software
Balmoral Software

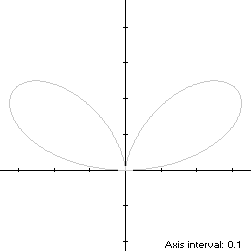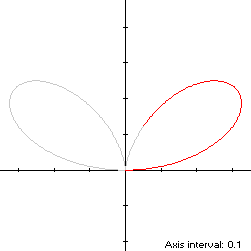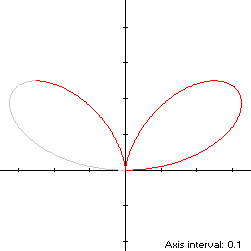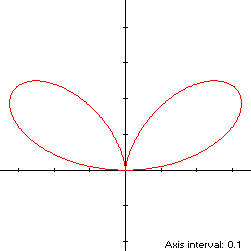
r(t) = sin(t)cos2(t), 0 ≤ t < πThe curve is traced out in a counterclockwise direction, starting from its minimum ordinate point at the origin when
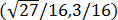 at
at
 which has an aspect ratio of
about 2.6.
which has an aspect ratio of
about 2.6.
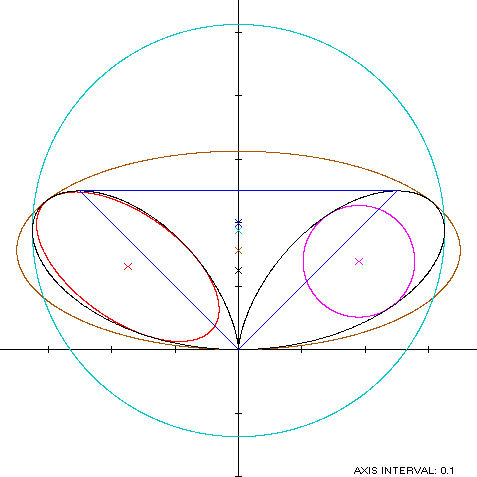
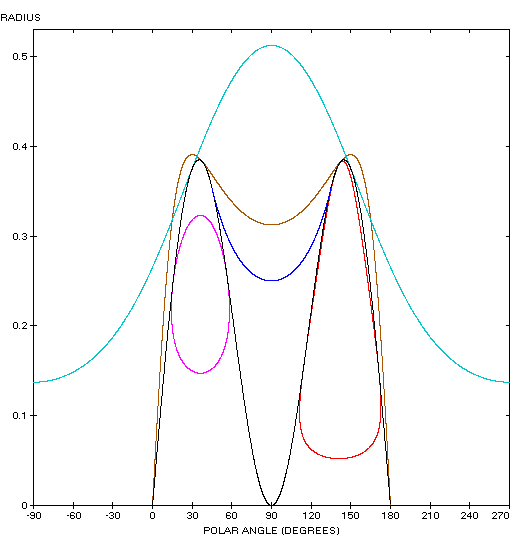
The lobes of the bifolium (red) are spread farther apart than those in the upper
half-plane of the comparably-sized four-leaved
rose
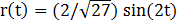 (black):
(black):
r'(t) = 3cos3(t) - 2cos(t),so by (L2), the perimeter of the convex hull is
which is about 14% shorter than that of the bifolium.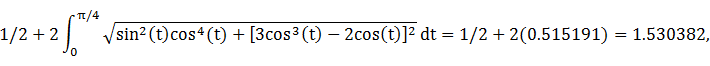
The line segment of the convex hull creates an isosceles triangle with the
origin, having an area of
By (C2), the associated centroid ordinate is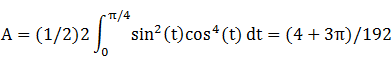
The convex hull component metrics can be summarized as follows: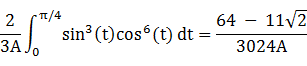
The area of the convex hull is 0.132421, about 1/3 larger than that of the bifolium. The centroid ordinate of the convex hull is the weighted average
Region Area Centroid ordinate Product Triangle 1/16 1/6 1/96 Remainder A = (4 + 3π)/192 Total (16 + 3π)/192
x(t) = r(t)cos(t) = sin(t)cos3(t)The radius of a circle centered on the y-axis and circumscribing the bifolium is at least its maximum abscissa, so a candidate for the circumcircle has radiusy(t) = r(t)sin(t) = sin2(t)cos2(t) = sin2(2t)/4
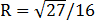 and center ordinate
and center ordinate
d/dt x(t)[y(t) - z] = d/dt sin3(t)cos5(t)has a zero at t* = Arccos(1/4)/2. The corresponding coordinates are
We then have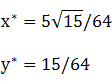
For verification,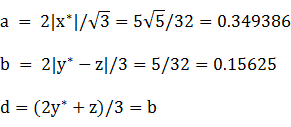
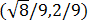 when
when
Since the bifolium is defined by a polar function, tR is also the clockwise rotation angle. The parametric coordinate functions of the rotated curve are: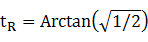
x(t) = r(t)cos(t - tR)The asymmetry can then be seen by comparing the upper half of the lobe (red) with a reflection of the lower half (blue):y(t) = r(t)sin(t - tR)
R = 0.087970
c = 0.189708
d = 0.139099
a = 0.165788To display the tilted ellipse in the left lobe for comparison with the incircle, we negate c and then rotate the ellipse clockwise by
b = 0.084029
c = 0.217709
d = 0.005909
*: With respect to rotated curve S
Figure Parameters Perimeter Area Centroid Incircle (lobe) R = 0.087970 0.552732 0.024312 (0.189708,0.139099) Inellipse (lobe) * a = 0.165788
b = 0.0840290.805983 0.043766 (0.217709,0.005909) Bifolium 1.788887 0.098175 (0,0.125) Convex hull 1.530382 0.132421 (0,0.199639) Circumellipse 1.646991 0.171504 (0,0.15625) Circumcircle R = 2.040524 0.331340 (0,0.1875)
 |  |
| Visitor from the planet Bifolium |
Copyright © 2021 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.