Lemniscate of Bernoulli

Lemniscate of Bernoulli LEMNISCATE OF BERNOULLI

Balmoral Software

The Lemniscate of Bernoulli, or two-leaved rose, is a bisymmetric figure-8 curve S with parametric equations

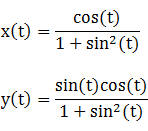
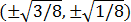for 0 ≤ t < 2π. As t increases from 0, S starts from its right-hand edge at (1,0), moves through Quadrant I to the origin at t = π/2, then clockwise through Quadrant III, its left edge (-1,0) at t = π, and Quadrant II before returning to the origin at t = 3π/2 and passing through Quadrant IV back to its starting point. The ordinate extrema of S are at

when

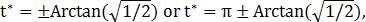so the width x height of its bounding rectangle is

Metrics

The perimeter of S is

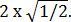
and its area is 1.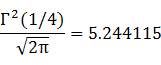

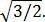Convex Hull

The convex hull is created by connecting the ordinate extrema points with two horizontal line segments of length

We have

so by (L1), the perimeter of the convex hull is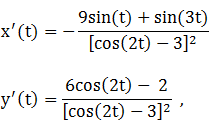

which is about 9% shorter than that of the lemniscate.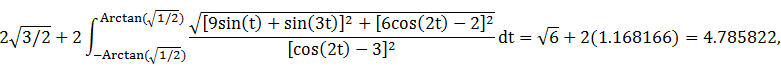

The line segments of the convex hull create a rectangle of area 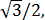as shown in blue in the left diagram below. By (A1), the area of the convex hull is

which is about 30% more than the area of the lemniscate.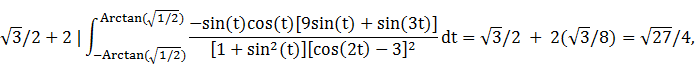

Circumcircle

The squared-distance function of S

is maximized at 1, so the circumradius is 1.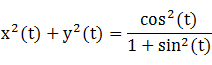

Circumellipse

From Lemma B, we have

This expression is maximized in the first quadrant at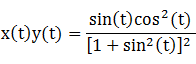

so the circumellipse dimensions are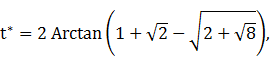

For verification, we have

Incircle (lobe)

Consider the right lobe of the lemniscate, where -π/2 ≤ t < π/2. The maximum radius of a circle centered on the x-axis and inscribed in the lobe is the maximum ordinate

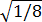of S, so a candidate for the lobe incircle has radius

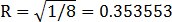and center abscissa

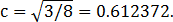The candidate circle must be contained within the lobe, so we require that c - R and c + R both be within the abscissa range [0,1] of the lobe, which is true. For verification, we have

Inellipse (lobe)

Using z = 1 in Lemma E,

has a zero at t* = 0.916671. The corresponding coordinates are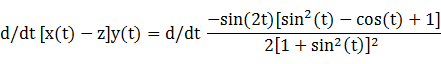

x* = 0.373344
y* = 0.296279

We then have

For verification,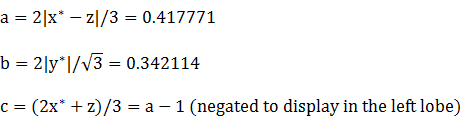

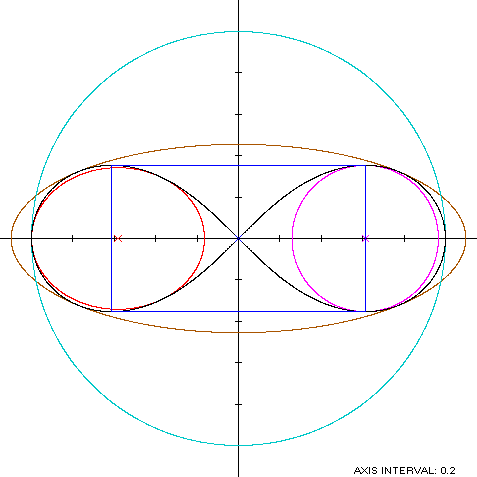
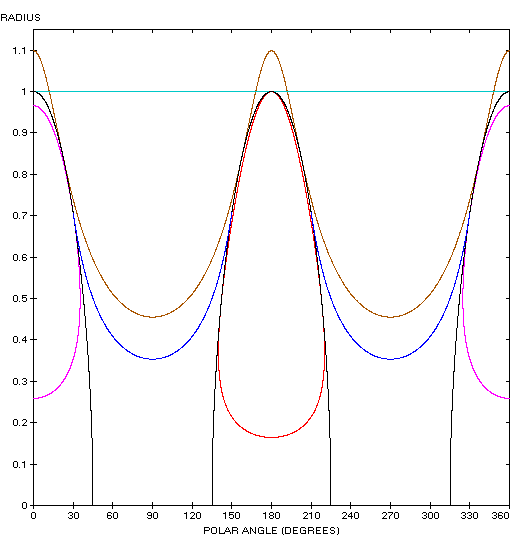
Summary Table

Figure Parameters Perimeter Area Centroid

Incircle (lobe) R = 2.221442 0.392699 (0.612372,0)q

Inellipse (lobe) a = 0.417771
b = 0.342114 2.393169 0.449013 (-0.582229,0)

Lemniscate of Bernoulli 5.244115 1

Convex hull 4.785822 1.299038

Circumellipse 5.093049 1.570797

Circumcircle R = 1 6.283185 3.141593

Figure	Parameters	Perimeter	Area	Centroid
Incircle (lobe)	R =	2.221442	0.392699	(0.612372,0)q
Inellipse (lobe)	a = 0.417771 b = 0.342114	2.393169	0.449013	(-0.582229,0)
Lemniscate of Bernoulli		5.244115	1
Convex hull		4.785822	1.299038
Circumellipse		5.093049	1.570797
Circumcircle	R = 1	6.283185	3.141593


Bug-eyed Space Jockey

The Lemniscate of Bernoulli (red) is a member of a group of figure-8 curves described on these pages, including (inside to outside) the dumbbell curve, the bowtie, the Lemniscate of Gerono and the dipole:

Top Page

Home