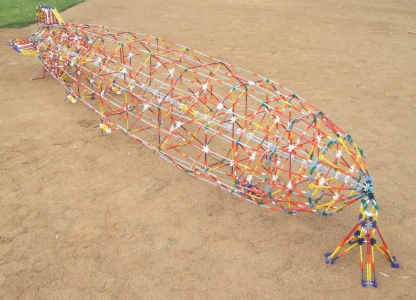
The Hindenburg

The Hindenburg

Here is a 1/54 scale model of the LZ 129 Hindenburg, the famous German zeppelin of the 1930's. This model of the airship is about 15 feet long and is made from nearly 5,000 K'NEX parts. Read on for more information on how this scale model was built. Click on the photos for larger versions.

Background
Digitizing
Basic Design
Frame Design Options
Optimum Frames
Longitudinal Stringers
Design Profile
Construction Plan
Fins
Mooring Tower
Engines & Control Car
Photo Gallery

1. Background

The giant airships of the 1930's provided swift and comfortable transoceanic passenger travel rivaling the ocean liners of the day, and the Hindenburg was one of the largest lighter-than-air flying machines ever built. With its rigid metal framework designed to hold huge cells of hydrogen or helium lifting gas, the Hindenburg is a good choice for scale modeling with the K'NEX construction toy. To create this model, we begin with David Fowler's excellent technical drawings of the Hindenburg at http://www.highriskadventures.com/airships. His side profiles of the zeppelin are particularly useful.

2. Digitizing

To begin, the zeppelin profile data needs to be digitized on the computer. One way to do this is to take screenshots of the airship profile pages in the PDF file at 100% size, then load them into Microsoft Paint. Zoom in to facilitate gathering pixel data points at various locations along the hull. This data can be normalized for a horizontal axis along the centerline of the airship (increasing towards the bow), with the zero point at the stern. Only data for the upper half of the airship needs to be collected, since the lower half is assumed to be symmetric (except for details such as the control car):


TABLE 1

Horizontal    Vertical    Location
  pixel        pixel
coordinate   coordinate

      0           0       Extrapolated stern endpoint
     75          40
    195          98
    314         137
    434         172       Base of fin (excluding rudder)
    531         198
    628         222
    726         245       Frame 33.5
    823         266
    920         286
   1017         304
   1126         321       Fin leading edge join
   1234         337
   1342         352       Frame 62 (1st gas vent)
   1451         365
   1559         376
   1667         387
   1776         396
   1788         397
   1884         403
   1992         411       Frame 92 (2nd gas vent)
   2101         417
   2209         424
   2317         429
   2436         434
   2555         437
   2674         440       Frame 123.5 (3rd gas vent)
   2793         443
   2913         446
   3031         446
   3151         446
   3270         446
   3388         446       Frame 156.5 (4th gas vent)
   3508         444
   3603         442
   3627         441
   3746         437
   3854         432
   3963         427
   4070         421       Frame 188 (5th gas vent)
   4179         414
   4288         405
   4395         394
   4504         380
   4612         363
   4720         342       Frame 218 (6th gas vent)
   4829         317
   4937         285
   5045         242
   5132         199
   5219         140
   5294          64       Frame 244.5
   5329          18
   5344           0       Nosecone

In this scale, the airship has a length of 5344 pixels and a maximum diameter of 2 x 446 = 892 pixels, and so it is almost exactly 6 times as long as its maximum diameter. These proportions are corroborated by the dimensions of the actual Hindenburg airship: length 804 feet and diameter 135 feet. A diagram of these data points serves to confirm the general shape of the airship profile (stern on the left):

3. Basic Design

Next, we need to determine the design possibilities in K'NEX for the airship. Sturdy, nearly-circular vertical frames over a range of different diameters are needed to simulate the curvature of the airship envelope. The longitudinal girders of the airship connect the perimeters of these vertical frames together, and require flexible or pivoting connections at some of the frames to allow for tapering of the outer envelope. A strong central longitudinal axis is also needed to hold everything together internally, and a simple way of creating that is with a long cylinder consisting of a series of white connectors with four parallel rods (typically grey ones). This design limits the center connections of the vertical frames to the four open spaces at right angles in the white connectors of the axis cylinder.

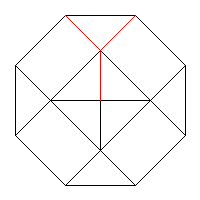
The cross-section of the actual zeppelin was a 36-sided regular polygon, but there are only a few possibilities for that shape in K'NEX without extensive bending of parts, particularly at smaller diameters. So we first tried a simpler 16-sided regular polygon (hexadecagon), and one way of creating it is to use two nested octagons with an offset of 22.5 degrees held in place with a ball-and-socket joint from one of the K'NEX figures:

However, this design proved to be unstable and irregular, and also had some challenges with attachment to the central axis using orange connectors. Instead, a sturdier solution is based on simple octagons with dual longitudinal stringers included on each of its sides, for a total of 16 longitudinal links between each vertical frame. While not as accurate as the actual zeppelin geometry, its overall shape and general appearance are achieved, as can be seen in the image at right.

4. Frame Design Options

Once the octagon is selected as the basic vertical frame shape, the next step is to determine the possibilities in K'NEX for a wide variety of frame sizes in order to properly simulate the streamlined airship shape. The octagon needs to connect rods to the central longitudinal axis at four points, so the resulting shape (with internal bracing) is:

Each side of the octagon is equivalent to a number g of green rods plus a number W of white rods, all linked by connectors (usually orange ones). For example, if the side of the octagon is a red rod connected with a yellow rod, the overall side length can be represented as g = 4 & W = 2. This is because a red rod is equivalent to four connected green rods, and a yellow rod is equivalent to two connected white rods. The octagon side must use an even value for g so that diagonal Y-shaped bracing can be provided as shown in red in the diagram above. The overall length S (in millimeters) of the octagon side between the centers of the holes of the connectors used for the vertices is

S = 37.6 g + 53.2 W mm

using known dimensions for the K'NEX parts.

What is the radius R (half the diameter) of an octagon created in this way? It is the distance from its center to one of its vertices, as shown in red in the diagram below:

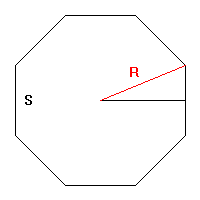
Since the interior angle of an octagon is 360/8 = 45 degrees, a right triangle can be visualized that has a central angle of 22.5 degrees and a height equal to one-half the octagon side S. Using a well-known formula from trigonometry, we have

R sin 22.5° = S/2

R = 1.3066 S

To provide enough detail for the airship appendages (engines, control car, etc.), the overall length of the model is 15 feet, or about a 1/54 scale with respect to the actual zeppelin. At the 6:1 aspect ratio described above, that scale results in a maximum diameter of 30 inches, or a maximum radius of 381 mm (at 25.4 mm per inch). Using the formula above and the previously-described requirement that the number g of green rods be even, we have 15 possibilities for the size of the vertical frames:


TABLE 2

----Frame side----   Radius

0 g   1 W    53 mm    70 mm
2 g   0 W    75 mm    98 mm
0 g   2 W   106 mm   139 mm
2 g   1 W   128 mm   168 mm
4 g   0 W   150 mm   197 mm
0 g   3 W   159 mm   209 mm
2 g   2 W   181 mm   237 mm
4 g   1 W   203 mm   266 mm
0 g   4 W   212 mm   278 mm
6 g   0 W   225 mm   295 mm
2 g   3 W   234 mm   307 mm
4 g   2 W   256 mm   336 mm
0 g   5 W   266 mm   348 mm
6 g   1 W   278 mm   364 mm
2 g   4 W   288 mm   376 mm

5. Optimum Frames

Next, we need to find the optimum collection of frames and the associated slant distances between their perimeters to simulate the outer shell of the zeppelin. The slant distance between the perimeters of adjacent frames can be somewhat larger than the horizontal distance between the frames since they are measured along the outer airship envelope where tapering occurs. Selection of the optimum set of frames is most easily done programmatically using the pixel profile data in Table 1 above. The maximum height in the profile data is 446 pixels, corresponding to the largest octagonal K'NEX frame in Table 2 with a radius of 376 mm, or a ratio of about 1.186 pixels per mm. With this ratio, we can convert the profile data in pixels to the corresponding number of millimeters, and then use that to find adjacent profile data points so that the given frame radius falls between them. For example, the smallest K'NEX frame in Table 2 has an octagonal side equivalent to one white rod with connectors, and a radius of 70 mm (83 pixels). That radius value falls between the second and third pixel profile data points in Table 1.

The first pass of the optimization process produces the following raw data, ordered from stern to bow:


TABLE 3

Frame    Slant distance   Error
side     to next frame

 53 mm        78 mm       -3 mm
 75 mm       140 mm        3 mm

106 mm       111 mm        2 mm
128 mm       123 mm        5 mm

150 mm        53 mm        0 mm
159 mm       135 mm       -6 mm

181 mm       167 mm        0 mm

203 mm        80 mm       -5 mm
212 mm       121 mm        7 mm
225 mm        99 mm        7 mm

234 mm       312 mm       -2 mm

256 mm       179 mm        2 mm
266 mm       303 mm       -1 mm

278 mm       447 mm        0 mm

288 mm       400 mm        0 mm

288 mm       397 mm       -2 mm

278 mm       283 mm       -3 mm
266 mm       133 mm       -4 mm

256 mm       216 mm       -3 mm

234 mm        64 mm      -10 mm
225 mm        81 mm       -5 mm
212 mm        52 mm        0 mm

203 mm        99 mm        7 mm
181 mm        76 mm        0 mm
159 mm        29 mm        8 mm

150 mm        65 mm        9 mm
128 mm        52 mm        1 mm
106 mm        65 mm       10 mm
 75 mm        39 mm       -1 mm
 53 mm

Table 3 lists the slant distances between frames if all of the possible K'NEX frame sizes were used for both bow and stern tapers of the airship. It also shows the errors resulting from the approximations of the actual slant distances with K'NEX parts. To avoid overcrowded frames and retain an accurate airship shape, the goal is to find optimal frames that are at least 8 inches (203 mm) apart, about the length of a grey K'NEX rod. This has the advantage of reducing error in the slant distances since they will be computed over a longer distance. Also, we want to ensure that frames close to the leading and trailing edges of the tail fins are included so that we have good attachment points. Those fin-attachment frames are:


TABLE 4

Horizontal    Vertical    Vertical   K'NEX    K'NEX    Location
coordinate   coordinate   distance   radius    side

  434 px       172 px      145 mm    139 mm   106 mm   Base of fin (excluding rudder)
 1126 px       321 px      271 mm    266 mm   203 mm   Fin leading edge join

There may be additional, intermediate frames that can also be used to anchor the fins.

Based on a manual analysis of the raw data in Table 3, optimal selections are grouped together to produce the following smaller list of frames and more-accurate slant distances:


TABLE 5

Frame    ---Slant distance---   Error
 side       to next frame

 53 mm   218 mm    3 g    2 W    1 mm
106 mm   233 mm    2 g    3 W    1 mm	Fin attachment point
150 mm   189 mm    5 g    0 W   -1 mm
181 mm   167 mm    3 g    1 W    0 mm
203 mm   300 mm    8 g    0 W    0 mm	Fin attachment point
234 mm   312 mm    4 g    3 W   -2 mm
256 mm   482 mm   10 g    2 W    0 mm
278 mm   447 mm    2 g    7 W    0 mm
288 mm   400 mm    5 g    4 W    0 mm
288 mm   397 mm    2 g    6 W   -2 mm
278 mm   416 mm    4 g    5 W    0 mm
256 mm   216 mm    0 g    4 W   -3 mm
234 mm   197 mm    1 g    3 W    0 mm
203 mm   204 mm    4 g    1 W    0 mm
150 mm   220 mm    3 g    2 W   -1 mm

Table 5 coincidentally contains 15 optimal frames, but is not related to the 15 possible K'NEX frame sizes in Table 2 since some frame sizes are reused at each end of the airship, and other sizes are not used at all. A diagram of these data points serves to confirm the general shape of the airship (the nose and stern cones will be fitted manually):

6. Longitudinal Stringers

Combining the information above, we can create a construction list for the airship frames and longitudinal stringers, beginning at the stern:


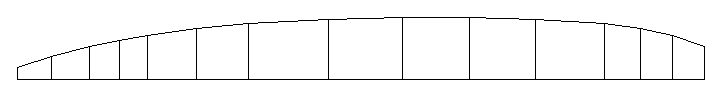
TABLE 6

Frame side   Diagonal bracing   Slant distance to next frame   Radius   Slant    Deviation from 180°

  W               g                       Y+B+g                 70 mm   218 mm           *
  Y               B                       Y+B+W                139 mm   233 mm           4 *
  R               Y                       R+g                  197 mm   189 mm           2 *
  Y+B             B+W                     B+W+g                237 mm   167 mm           2
  R+W             Y+g                     2R                   266 mm   300 mm           2
  Y+B+W           B+W+g                   R+Y+W                307 mm   312 mm           3
  R+Y             Y+B                     2R+Y+B               336 mm   482 mm           2
  R+B+W           Y+W+g                   G+Y+B+W              364 mm   447 mm           2
  G+B             R+W                     G+R+g                376 mm   400 mm           2
  G+B             R+W                     G+Y+B                376 mm   397 mm           2
  R+B+W           Y+W+g                   G+R+W                364 mm   416 mm           2
  R+Y             Y+B                     G                    336 mm   216 mm           4
  Y+B+W           B+W+g                   Y+W+g                307 mm   197 mm           4
  R+W             Y+g                     R+W                  266 mm   204 mm           8 *
  R               Y                       Y+B+g                197 mm   220 mm           *

where the following symbols are used:


	g: Green rod
	W: White rod
	B: Blue rod
	Y: Yellow rod
	R: Red rod
	G: Grey rod
	+: Orange connector

The rods in the diagonal bracing are one K'NEX size down from those in the frame side. Table 6 also includes calculations for how closely to a straight angle (180°) the longitudinal stringers intersect the perimeters of the vertical frames. For example, in the side-view profile below, the frame with one yellow rod for each side (radius 139 mm) has the following dimensions (mm), with respect to its two adjacent frames:

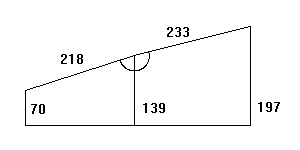
These dimensions result in a calculation of 176° for the indicated angle, or about 4° off of a straight angle, using the inverse-cosine trigonometric function:


	        139 - 70            139 - 197
	Arccos ---------- + Arccos ----------- = 176°
	          218                  233

As described above, the zeppelin model has 16 stringers connected to the perimeter of each octagonal frame. For the best uniform appearance, these stringers pass through the corners of the octagons, as well as through the midpoints of their sides. The corner stringers pass through the central hole of each green connector used for the frame corner. In practice, depending on the stringer lengths involved, a deviation of more than about 4° or so from a straight 180° intersection angle results in too much tilting of the green connector and associated distortion of the vertical frame and/or stringers. Therefore, the stringer connections at the frames indicated with asterisks in Table 6 (along with the nose and tail cones) have pivoting attachments to allow for steeper angles between frames.

At those locations needing flexible angles between consecutive frames, stringers are attached to the frame perimeter with pivots. These pivots can be realized with dark-grey K'NEX connectors that attach to the end of stringer rods and swing freely on the octagon sides. Multiple pivots may be needed on each side, depending on the number of fore and aft stringer connections.

7. Design Profile

To verify the overall K'NEX design plan prior to actual construction, a simple side-view profile can be created using Table 6. Each of 15 half-frame profiles is constructed with a dark-grey connector at the bottom, representing its connection to the horizontal central axis. The appropriate sequence of rods and orange connectors is used for the inner octagon bracing up to a white connector, then another sequence (equal to half the octagon side) for the distance spanned by the Y-shaped diagonal bracing to the outer perimeter of the frame:

In Table 6, frames not terminated with an asterisk at the end of the line are located where there isn't much tapering in the airship envelope, and so stringer pivots do not not need to be used on them. In this case, the stringer rods between the frames can be rigidly attached to connectors on the frame structure. This design is shown in the right-hand profile photo below, while the left-hand photo shows the pivots used when stringers make a slight corner at the frame:

8. Construction Plan

Now looking at the connections of longitudinal stringers to the octagonal frame sides, we can create a blueprint for construction of the airship frames. In locations where stringers run nearly parallel with the central axis, a white or yellow connector can be used to connect them at the midpoint of the octagon side if that side is divisible in half (containing the equivalent of an even number of white pieces). In the other cases where an octagon side contains an odd number of white pieces, an orange connector can be used with spacers on a central white rod. Finally, to avoid "bowing" in the stringers, some additional stringer passthroughs were used instead of connectors at side midpoints, like those used at the frame corners.

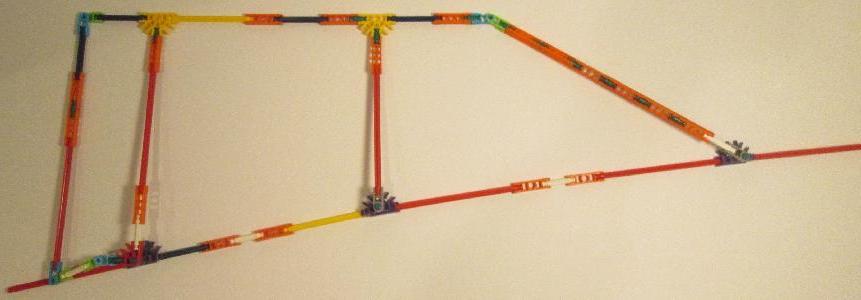
Starting at the stern and moving forward, a frame construction plan is as follows:


TABLE 7

Frame side     Implementation   Pattern                                    Photo            Notes

W              W                XX|                                        Frame style A
Y              W+W              CX|(YYYY)|XC                               Frame style B
Y (at fin)     Y                CXFCCXCCFXC                                Frame style Bf
R              B+B              CC|X|CC(YYYY)CC|X|CC                       Frame style C    Start of stringers at octagon corners
R (at fin)     R                CCXCC|FCCXCCF|CCXCC                        Frame style Cf   Start of stringers at octagon corners
Y+B            g+W+W+g          (OOOO)..:(WWWW):..(OOOO)                   Frame style D
R+W            B+W+B            (BBBB)(OOOO)|OC(OOOO)(BBBB)                Frame style E
R+W (at fin)   R+W              CCCCCCCCCCFCCOCCFC(OOOO):..                Frame style Ef
Y+B+W          g+W+W+W+g        (OOOO)..:(OOOO)|OC(OOOO):..(OOOO)          Frame style F
R+Y            W+B+B+W          ..:(OOOO)(BBBB)(OOOO)(BBBB)(OOOO):..       Frame style G    Stringer passthrough at midpoint
R+B+W          g+B+W+B+g        (OOOO)(BBBB)(OOOO)|OC(OOOO)(BBBB)(OOOO)    Frame style H
G+B            Y+g+g+Y          (YYYYYYYYY)(OOOO)(OOOO)(OOOO)(YYYYYYYYY)   Frame style I    Stringer passthrough at midpoint
G+B            Y+g+g+Y          (YYYYYYYYY)(OOOO)(OOOO)(OOOO)(YYYYYYYYY)   Frame style I    Stringer passthrough at midpoint
R+B+W          g+B+W+B+g        (OOOO)(BBBB)(OOOO)|OC(OOOO)(BBBB)(OOOO)    Frame style H
R+Y            W+B+B+W          ..:(OOOO)(BBBB)(WWWW)(BBBB)(OOOO):..       Frame style J
Y+B+W          g+W+W+W+g        (OOOO)..:(OOOO)|OC(OOOO):..(OOOO)          Frame style F
R+W            B+W+B            CCCC|X|(OOOO)|XC(OOOO)|X|CCCC              Frame style K    End of stringers at octagon corners
R              B+B              CC|X|CC(YYYY)CC|X|CC                       Frame style L

where the following notation is used:


	g: Green rod
	W: White rod (inline space for 2.5 connectors)
	B: Blue rod (inline space for 6 connectors)
	Y: Yellow rod (inline space for 11 connectors)
	R: Red rod (inline space for 18 connectors)
	G: Grey rod (inline space for 28 connectors)
	+: Orange connector inline (occupies space of 6 connectors)

	X: Dark-grey connector at end of stringer
	O: Orange connector at end of stringers
	F: Fin connector
	C: Dark-grey connector as spacer (rotating freely)
	|: Light blue washer (1/2 connector thickness)
	.: Connector space on white rod
	:: Washer space on white rod
	(OOOO): Orange connector (connected inline with rods)
	(YYYY): Yellow connector
	(WWWW): White connector
	(BBBB): Blue rod
	(YYYYYYYYY): Yellow rod

9. Fins

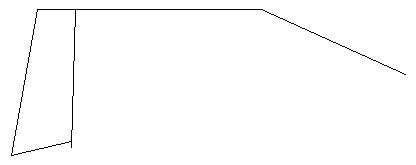
Using the same digitizing methods described above, the pixel coordinates of the fin can be calculated in Paint. These calculations result in the following fin profile:

With the aforementioned ratio of 1.186 pixels per mm, we can determine the K'NEX parts to approximate the fin profile:

Based on the technical drawings referenced above, the maximum thickness of each fin is 89 pixels, or about 75 mm at the scale of the model. The average fin thickness is somewhere around half that, equivalent to two connectors attached by a single green rod. Therefore, two sides of each three-dimensional fin are created and connected together with a green rod. A total of three connections of each fin to the main body of the airship model are determined by fitting. Technically, the lower fin of the actual airship is slightly different from the other three in that it tapers upward slightly towards the stern. However, this minor change is not implemented in the K'NEX model, and all four of its fins are identical.

The following pictures highlight the connections of the fin to the airship envelope:

10. Mooring Tower

Photos of the mooring mast used by the Hindenburg at Lakehurst were employed as a guideline for its design in K'NEX. Assuming the moored airship model is nearly resting on the ground, flat on its longest frame side, then the height of the mooring mast is equivalent to the distance between the center axis of the airship and the side of its largest frame. This distance is slightly (about 8%) shorter than the radius of the largest frame, since we are measuring to the midpoint of the side of the octagon resting on the ground rather than to one of its vertices. In the geometry of the K'NEX model, the height of the mooring mast is the length of the largest frame's diagonal bracing plus one-half of its octagon side, or

4g + W + (2g + 4W)/2 = 5g + 3W = 348 mm.

This size provides a number of options for a realistic mast design. In particular, outer supporting struts at a steeper angle of about 60 degrees are possible, similar to the actual Lakehurst mooring mast. We ended up extending the height of the mooring mast by one additional green rod to facilitate the mast design and allow clearance for the control car.

11. Engines & Control Car

Again using the same digitizing method above, scale approximations to the engines and control car are created using K'NEX parts. These are positioned on the airship model according to the technical drawings. Here are some close-ups of the engine pods:

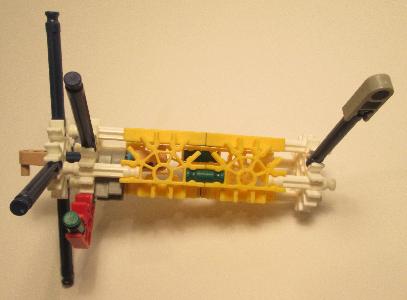
And here are some close-ups of the control car:

A couple of small artificial props can be seen in some of the photos; these are added to prevent the model from sagging or tipping since its central axis is somewhat flexible.

12. Photo Gallery

Close-ups of the airship-mooring mast connection:

A rear quarter view of the airship model:

A modification of the central axial tube can be used to allow the airship model to be easily disassembled into sections:

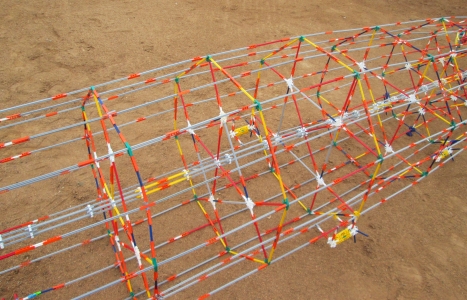
If the K'NEX airship were the actual size of the Hindenburg zeppelin, our 1/54 model would be the size of a yellow rod in comparison (outlined in red below):


HINDENBURG AIRSHIP PART COUNTS

Orange Connector        1007
Green Rod                622
Dark Grey Connector      500
White Rod                468
Blue Rod                 399
Yellow Rod               289
Grey Rod                 243
Red Rod                  227
Purple Connector         204
White Connector          184
Washer                   169
Green Connector          144
Yellow Connector         140
Red Connector             59
Hinge                     50
Brown Connector           29
Blue Connector            16
Light Grey Connector       2

Total                   4752