Equable Spherical Wedge

EQUABLE SPHERICAL WEDGE

Balmoral Software

Solutions: 4

A spherical wedge is defined by two semicircular faces sharing a diameter of a sphere as a common edge; a real-world example is a segment of an orange. The wedge is bounded by the semicircles and a portion of the surface of the sphere known as a spherical lune, for its crescent or moon-like shape:

The angle defining the wedge is in a plane normal to the diameter shared by its sides; let it be a fraction a of a full circle, 0 < a < 1. Then the volume and lune surface area of the wedge are the corresponding fractions of a sphere of radius r:

V = (4/3)r³a π
S = 4r²a π + 2 semicircular sides = 4r²a π + r² π

The lune area 4r²a π and the semicircle area r² π/2 are integer multiples of π. By equability,

V = S
(4r - 12)a = 3

If r = 3, then the semicircle area divided by π is r²/2 = 9/2, which is not an integer, so r ≠ 3 and we can write

Since r²/2 is an integer, r is the square root of an even integer:

for some integer k. The lune area divided by π is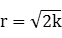

which is irrational unless r is an integer. We then have

Since 4r²a and r are integers, r - 3 divides 27, and therefore r ∈ {4,6,12,30}. The four solutions are: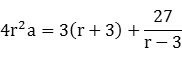

r a Angle Semicircle area Lune area V=S

4 3/4 270° 8 π 48 π 64 π

6 1/4 90° 18 π 36 π 72 π

12 1/12 30° 72 π 48 π 192 π

30 1/36 10° 450 π 100 π 1000 π

r	a	Angle	Semicircle area	Lune area	V=S
4	3/4	270°	8 π	48 π	64 π
6	1/4	90°	18 π	36 π	72 π
12	1/12	30°	72 π	48 π	192 π
30	1/36	10°	450 π	100 π	1000 π

Top Page

Home