Balmoral Software
|
Solutions: 6 (integer dimensions) Solutions: 3 (non-integer dimensions, conjectured) |
There are exactly 6 equable trirectangular tetrahedra with integer dimensions. It is conjectured that no more than 3 additional equable trirectangular tetrahedra have non-integer dimensions.
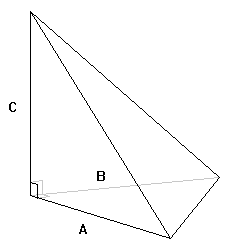
Let the lengths of the three orthogonal edges meeting at the corner be A, B and C; these are referred to as the dimensions of the tetrahedron. We begin by showing that the dimensions are rational. The volume of the tetrahedron and the areas of the three non-base faces are integers by convention:
ABC/6, AB/2, AC/2, BC/2 ∈ ℤIt follows that
is rational, and analogously for B and C. Therefore, we can express A, B and C in terms of their lowest common denominator d as a/d, b/d and c/d, where a, b, c and d are positive integers. Without loss of generality, we may take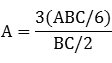
a ≥ b ≥ c ≥ 1Equating volume V and surface area S, we have
There can be no equable solution when the shortest edge length c/d ≥ 15:
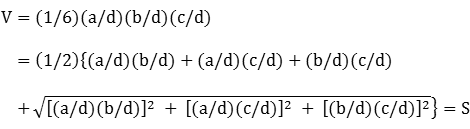
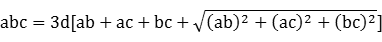
[1]
which shows that [1] is not satisfied since the volume exceeds the surface area. Next, assume that c ≤ 6d. Then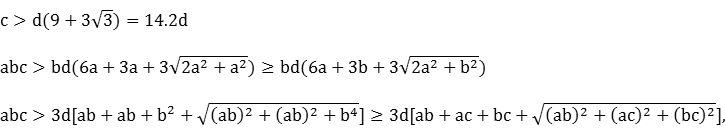
so again [1] is not satisfied. Therefore, all equable solutions occur when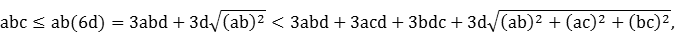
6d + 1 ≤ c ≤ 15d - 1Assume the above inequality holds, and let b > 12d(15d - 1). Then
[a - 6d(15d - 1)][b - 6d(15d - 1)] ≥ [b - 6d(15d - 1)]2 > [6d(15d - 1)]2and it follows that
abc ≥ ab(6d + 1) > 6abd + 6ad(15d - 1) + 6bd(15d - 1) ≥ 6abd + 6acd + 6bcdSince= 3d(ab + ac + bc) + 3abd + 3acd + 3bcd
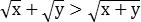 for positive x and y, we have
for positive x and y, we have
which shows that [1] is not satisfied. Therefore, all equable solutions occur when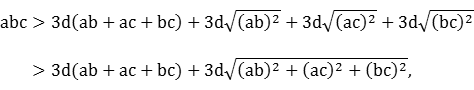
Dividing both sides of [1] by 3abcd, we have
6d + 1 ≤ c ≤ 15d - 1 and c ≤ b ≤ 12d(15d - 1) [2]
The left side must be positive for an equable solution. Squaring both sides reduces to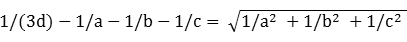
Since b ≥ c ≥ 6d + 1, the right side is positive. The condition
[(b - 6d)(c - 6d) - 18d2]a = 6d(b - 3d)(c - 3d) - 54d3 [3]
must hold so that division in [3] produces a positive value for the edge length numerator a:
(b - 6d)(c - 6d) > 18d2 [4]
By convention, the lateral face areas ab/(2d2), ac/(2d2), bc/(2d2) and the volume abc/(6d3) must all be integers. By subtraction, the base area is also an integer since the total surface area is an integer.
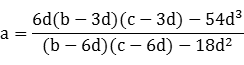
[5]
For a fixed value of d, it is a simple matter to evaluate the
(9d - 1)(360d2 - 45d + 2)/2feasible values of (b,c) in [2] to find those for which:
d A B C Face AB/2 Face AC/2 Face BC/2 Base V=S 1 18 14 12 126 108 84 186 504 1 24 12 12 144 144 72 216 576 3 36 12 32/3 216 192 64 296 768 1 32 24 8 384 128 96 416 1024 1 54 12 10 324 270 60 426 1080 1 66 18 8 594 264 72 654 1584 3 144 12 28/3 864 672 56 1096 2688 1 168 16 8 1344 672 64 1504 3584 3 336 36 20/3 6048 1120 120 6152 13440
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.