Balmoral Software
Solutions: 0
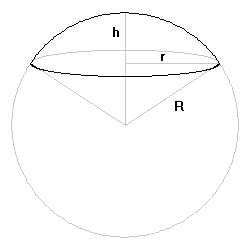
Let R be the radius of a sphere and h the height of a spherical segment of one base, where 0 < h < 2R. The volume of the spherical segment is V, where
V/π = h2(R - h/3)The lateral surface area of the spherical segment is Sl, where
Sl/π = 2RhThe surface area of the base is Sb, where
Sb/π = r2and r is the radius of the base. The above three quantities are integers by convention. The base radius is related to the sphere radius and segment height by
r2 = R2 - |R - h|2 = 2Rh - h2 = Sb/π,so
Sl/π - Sb/π = h2is an integer and therefore
is rational. Then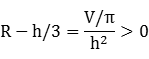
Sl/π - 2h2/3 and 2(R - h/3)are both rational, so
is also rational, and it follows that h is an integer.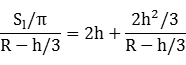
The equability requirement is
V/π = Sl/π + Sb/πh2(R - h/3) = 2Rh + 2Rh - h2
We have
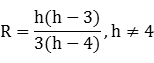
[1]
so h = 5. It follows from [1] that R = 10/3. But then Sb/π = 25/3 is not an integer, so there are no equable spherical segments with integer volume and surface area sections.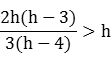
4 < h < 6,
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.