Balmoral Software
Solutions: 3
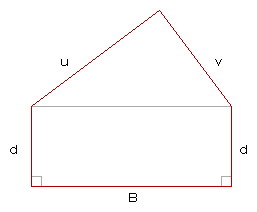
A rectangular pentagon can be thought of as an integer-sided convex pentagon with the added restriction that two non-adjacent sides have equal lengths, each of which forms a right angle with the intervening side.
The notation (Base,Side,Side,Height) = (B,u,v,d) will be used to describe the rectangular pentagon. The sides u and v of the triangular portion can be swapped without changing the area or perimeter of the pentagon, so we won't consider these to be separate solutions and can take u ≥ v.
There are exactly 3 equable rectangular pentagons.
Proof. For a convex rectangular pentagon, the base angles of the triangular portion must be acute. Thus, if the triangle is obtuse or right, then only its longest side can be the base.
By convention, the area of an equable rectangular pentagon is an integer, as is that of its rectangular portion, so by subtraction the triangle is Heronian. For an equable rectangular pentagon, we have
where AT and PT are the integer area and integer perimeter of the triangular portion. Since each side of a Heronian triangle must be at least 3, we have B ≥ 3 and can solve [1] for d:
A = AT + Bd = PT + 2d = P, [1]
Since d ≥ 1, we have
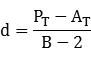
[2]
PT - AT ≥ B - 2 ≥ 1,so the triangle is perimeter-dominant, and we can write
Referring to results on Heronian perimeter-dominant triangles, we now consider each of these triangles as a candidate for the upper portion of an equable rectangular pentagon.
B ≤ PT - AT + 2 [3]
Case 1: (6,5,5) triangle
PT = 16By [3], a solution for d can exist only when
AT = 12
B ≤ 16 - 12 + 2 = 6,which is satisfied when any of the triangle sides is the base. However, by [2], only a base of 6 results in an integer height d:
The single result is the (6,5,5,1) rectangular pentagon.
B d 6 4/(6 - 2) = 1 5 4/(5 - 2) = 4/3
Case 2: (8,5,5) triangle
PT = 18Since this triangle is obtuse, only the side of length 8 can be the base, and by [2],
AT = 12
The single result is the (8,5,5,1) rectangular pentagon.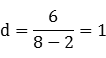
Case 3a: (a,a-1,3) triangles, a ≥ 3
By results presented elsewhere, these triangles are not acute, so by [3], we have
The only feasible solution in the Case 3a results table is the (5,4,3) triangle with B = a = 5, PT = 12 and AT = 6, so by [2],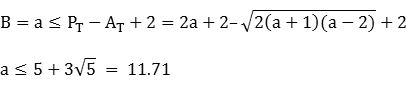
The single result is the (5,4,3,2) rectangular pentagon.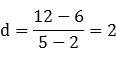
Case 3b: (a,a-2,4) triangles, a ≥ 4
Again, these triangles are not acute, so by [3], we have
The only feasible solution in the Case 3b results table again is the (5,4,3) triangle.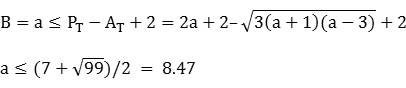
Summary of equable rectangular pentagons:
Base,side,side,height Perimeter=Area (5,4,3,2) 16 (6,5,5,1) 18 (8,5,5,1) 20
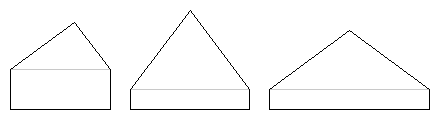
One of the three equable rectangular pentagons produces an equable solid of revolution by rotating it around its base. Let hT be the height of the triangular portion of the pentagon, so that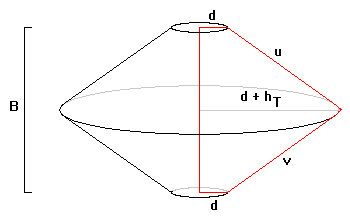
hT = 2AT/BThen the solid of revolution is two back-to-back conical frustums, each defined by radii d and
V/π = B[d2 + d(d + hT) + (d + hT)2]/3 = d2B + 2dAT + 4AT2/(3B)Evaluating these formulas for the three equable rectangular pentagons, the (6,5,5,1) one gives rise to an equable solid with V = S = 62π:S/π = (d + d + hT)(u + v) + 2d2 = 2(d + AT/B)(u + v) + 2d2
B u v d AT V S 5 4 3 2 6 268π/5 264π/5 6 5 5 1 12 62π 62π 8 5 5 1 12 56π 52π
B, u, v and d are positive integers.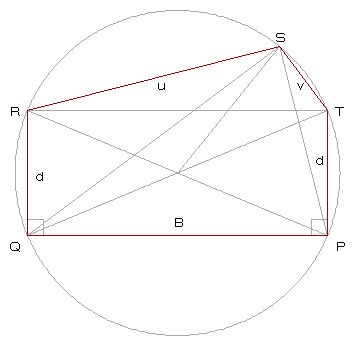
The four corners of the rectangular portion of a cyclic rectangular pentagon (B,u,v,d) are co-circular, so the pentagon's circumcenter is the centroid of the rectangle, which is the intersection of the two diagonals of the rectangle. Therefore, the ascending diagonal QT of the rectangle is a diameter D of the circumcircle, which forms a right triangle PQT with the base B and the right side d:
Since B and d are integers, so is D2.
B2 + d2 = D2 [4]
Consider the two radii of length D/2 connecting the circumcenter to the endpoints R and S of the upper-left side u. By the Triangle Inequality,
v ≤ u < D/2 + D/2 = DNext, consider the cyclic quadrilateral QRST that is contained in a semicircle. Since QST is a right triangle, the diagonals of this quadrilateral are
Applying Ptolemy's Theorem, we have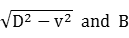
Using [4], this reduces to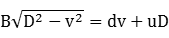
Since D2 is an integer, the left side of [5] is an integer, and so D is rational. It follows that D is an integer.
D4 - (d2 + u2 + v2)D2 = 2duvD [5] D3 - (d2 + u2 + v2)D - 2duv = 0 [6]
At this point, we can establish that none of the three equable rectangular pentagons satisfies [4], so they are not cyclic.
The circumcircle of triangle RST is the same as that of the aforementioned quadrilateral. By the SSS Theorem, the circumradius D/2 is related to the triangle area AT by:
so AT is rational. By [1], the cyclic rectangular pentagon has rational area, and so it is a Robbins pentagon. It follows from Lemma 5 of [A] that the rectangular pentagon area is an integer, and triangle RST is therefore Heronian. By Theorem 8 of [A], all of the diagonals of the cyclic rectangular pentagon are rational since three of them are the integer diameter D and the upper triangle base B. It follows that the remaining diagonals
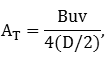
[7]
are rational, and by the previous reasoning, are integers. Therefore, the area and diagonals of a cyclic integer-sided rectangular pentagon are all integers.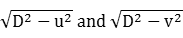
Cyclic rectangular pentagons may be found by searching over the following domain:
A = B[d + uv/(2D)]The height of the pentagon is
h = d + 2AT/B = d + uv/D,so its height/width aspect ratio is
Following is a list of the 22 cyclic rectangular pentagons with sides not exceeding 100, sorted by diameter and area: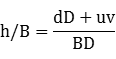
The corresponding diagrams can be viewed here.
B u v d Circumcircle
diameter DArea Perimeter P-S
diagonalQ-S
diagonal24 15 15 7 25 276 68 20 20 20 15 7 15 25 342 72 20 24 48 30 30 14 50 1104 136 40 40 40 30 14 30 50 1368 144 40 48 63 52 25 16 65 1638 172 39 60 60 52 16 25 65 1884 178 39 63 60 39 33 25 65 2094 182 52 56 39 25 16 52 65 2148 184 60 63 56 39 25 33 65 2268 186 52 60 52 33 25 39 65 2358 188 56 60 72 45 45 21 75 2484 204 60 60 60 45 21 45 75 3078 216 60 72 84 68 40 13 85 2436 218 51 75 75 68 13 40 85 3390 236 51 84 51 40 13 68 85 3624 240 75 84 77 51 40 36 85 3696 240 68 75 75 51 36 40 85 3810 242 68 77 68 40 36 51 85 4044 246 75 77 96 60 60 28 100 4416 272 80 80 80 60 28 60 100 5472 288 80 96 75 44 35 100 125 7962 354 117 120 100 75 35 75 125 8550 360 100 120
The 889 cyclic rectangular pentagons with sides not exceeding 1000 are listed here, sorted by diameter and area.
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.