Balmoral Software
Solutions: 1
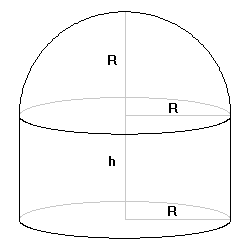
Consider the "hemispherical cylinder" consisting of a hemisphere of radius R surmounting a cylinder of the same radius and of height h. There is one such polytope that is equable, with integer-valued surface area sections.
The total volume of the hemisphere and cylinder is V, where
The surface area of the hemisphere is Sh, where
V/π = (2/3)R3 + R2h [1]
The lateral surface area of the cylinder is Sc, where
Sh/π = 2R2 [2]
The surface area of the cylinder base is Sb, where
Sc/π = 2Rh [3]
The above four quantities are integers by convention. From [1], [2] and [3], we have
Sb/π = R2 [4]
so R is rational. By [4], R2 is an integer, and it follows that R is an integer. The equability requirement is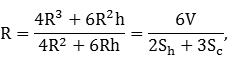
which reduces to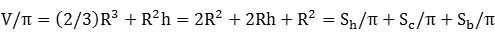
The numerator and denominator must have the same sign, so
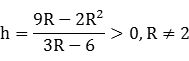
[5]
2 < R < 9/2In the latter case, h = 2/3 by [5] but Sc/π = 2Rh = 16/3 is not an integer. However, if R = 3, then h = 3 and 2Rh = 18. Therefore, the only equable hemispherical cylinder has a radius and height of 3, and volume and surface area equal to 45 π. It is a solid of revolution of a non-equable closed shape consisting of aR = 3 or R = 4
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.