Balmoral Software
Solutions: 3
(6, 8 or 12 faces)
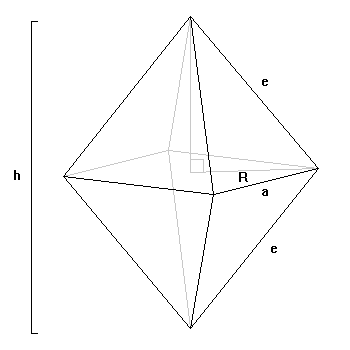
A dipyramid is created from two identical right pyramids, each of which has a base that is a regular polygon of n sides of length a, joined base-to-base. The height of the entire dipyramid is h. The area of the regular polygon is
Bn = (n/4)a2cot(180°/n)The volume of the dipyramid is twice that of the pyramid:
and is assumed to be an integer by convention. The 2n faces of the dipyramid are identical isosceles triangles, each of which has a base of length a and two sides of length e, where
V = 2(1/3)(h/2)Bn = (n/12)a2hcot(180°/n) [1]
e2 = (h/2)2 + R2and R is the circumradius of the regular polygon:
The area of each triangle then is
R = (a/2)csc(180°/n) [2]
which is also assumed to be an integer by convention. By equability,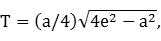
which reduces to
V = 2nT = S, [3]
Substituting [4] in [1] and [3], we have
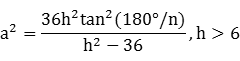
[4]
where the real number cn is defined as
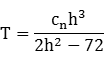
[5]
Some values of cn are:
cn = 3tan(180°/n) [6]
For h > 6, T has a minimum value of
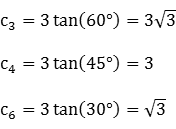
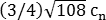at h =
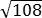, and two real solutions for h exist for each integer value
of T above this minimum. Therefore, there are infinitely-many equable isosceles
dipyramids.
We henceforth restrict the analysis to the more distinctive cases where
cn is the square root of an integer, which occurs only when
n = 3, 4 or 6. We can see from [5] and [7] that T can be an integer only
when h is an integer (n = 4), or h is an integer multiple of 
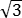(n = 3 or 6).
Case n = 4
In the first case, we have
so the fraction on the right is a positive integer, and therefore its numerator is at least as large as its denominator:
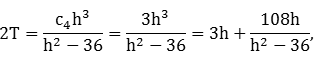
[8]
108h ≥ h2 - 36We can iterate [8] over the range 7 ≤ h ≤ 108 to find all solutions for which 2T is an even integer; the single result is
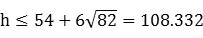
h = 12, T = 24
Case n = 3
Now let h =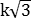 
for some positive integer k. For n = 3, we have
As before,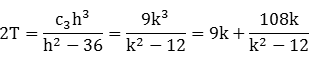
108k ≥ k2 - 12If h > 6, then k >
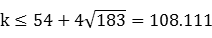
= 3.464, so we iterate over the range 4
≤ k ≤ 108 to find the solution
k = 4, h =, T = 72
Case n = 6
Similarly, we have for n = 6:
We can then iterate over the range 4 ≤ k ≤ 36 to find the solution
36k ≥ k2 - 12
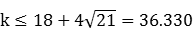
k = 4, h =It follows from [4] and [6] that
and from [2] that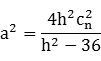
R2 = (a2/4)(1 + 9/cn2)
Summary
The three equable solutions are:
Both the equable octahedron and the equable dodecahedron have identically-sized faces.
n cn h a R e T V = S 3 12 72 432 4 3 12 24 192 6 24 288
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.
