Equable Cone

EQUABLE CONE

Balmoral Software

Solutions: 10

For a right circular cone with base radius r and height h, we have

which reduces to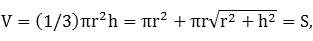

Equivalently,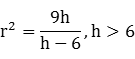

The base area must be an integer multiple of π, so let r² = k for some positive integer k. We then have

so h is rational. The volume V must also be an integer multiple of π, and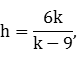

Therefore, k - 9 must divide 162, and so r² ∈ {10,11,12,15,18,27,36,63,90,171}. The following are all 10 equable right cones, ordered by volume: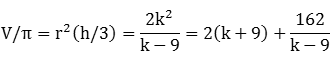

h r² r Lateral surface area/π V/π=S/π

12 18 4.243 54 72

15 15 3.873 60 75

9 27 5.196 54 81

8 36 6 6.000 60 96

24 12 3.464 84 96

33 11 3.317 110 121

7 63 7.937 84 147

20/3 90 9.487 110 200

60 10 3.162 190 200

19/3 171 13.077 190 361

h	r²	r	Lateral surface area/π	V/π=S/π
12	18		4.243	54	72
15	15		3.873	60	75
9	27		5.196	54	81
8	36	6	6.000	60	96
24	12		3.464	84	96
33	11		3.317	110	121
7	63		7.937	84	147
20/3	90		9.487	110	200
60	10		3.162	190	200
19/3	171		13.077	190	361

The only equable solution where r is also rational is the all-integer solution h = 8 and r = 6, for which V = S = 96 π. That cone is a solid of revolution of an equable right triangle with base 6 and height 8, having perimeter and area equal to 24.

Top Page

Home