Balmoral Software
Solutions: 0
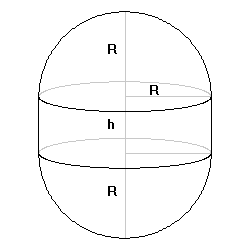
Consider the capsule consisting of two hemispheres of radius R adjoining a cylinder of the same radius and height h. The total volume of the polytope is V, where
V/π = Vsphere/π + Vcylinder/π = (4/3)R3 + R2hThe surface area of each hemisphere is Shemi, where
Shemi/π = 2R2The lateral surface area of the cylinder is Scyl, where
Scyl/π = 2RhThe above three quantities are integers by convention. We can write
so R is rational. The equability assumption is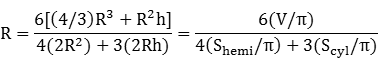
V = 2Shemi + Scyl,which reduces to
3h(R - 2) = 4R(3 - R)Incidentally, h is rational since it can be written as the quotient of rationals if R ≠ 2. Since h > 0 and R > 0, R - 2 and 3 - R must have the same sign, so
2 < R < 3By writing the fractionShemi/π = 2R2 ∈ {9,10,11,12,13,14,15,16,17}
4R2 ∈ {18,20,22,24,26,28,30,32,34}
we can see that no feasible value of 4R2 produces an integer value of the numerator, so R cannot be rational, a contradiction. Therefore, there are no equable capsules with integer-valued volume and surface area sections.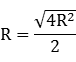
The capsule is a solid of revolution of a stadium with radius R and height h. That stadium is itself equable for any h when R = 2, having area and perimeter equal to 4(h + π).
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.