Balmoral Software
Solutions: 1 (rational height)
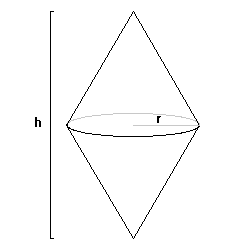
A bicone is a solid of revolution created by rotating a rhombus around one of its diagonals. There is exactly one equable bicone with a rational height and rational square of radius.
Proof. For an equable bicone with radius r and overall height h, we have
which reduces to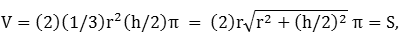
for an equable solution. By convention, W = V/π = r2h/3 is a positive integer. Then r2 = 3W/h > 9, so h < W/3 and [1] can be written
(r2 - 9)h2 = 36r2, r > 3 [1]
(3W/h - 9)h2 = 36(3W/h)The equable solutions for h are the zeroes of the cubic3h3 - Wh2 + 36W = 0, 0 < h < W/3
fW(h) = 3h3 - Wh2 + 36WSince
d/dh fW(h) = 9h2 - 2Whand
d2/dh2 fW(h) = 18h - 2W,fW has critical points at h = 0 and h = 2W/9 > 0. The first is a relative maximum and the second a relative minimum, and fW(0) = 36W > 0. Therefore, there are two positive real zeroes when
In conclusion, there are infinitely-many equable bicones with real dimensions, and volumes that are integers of at least 47, times π.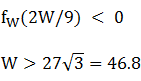
and any equable solution requires that h > 6. It follows that
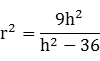
[2]
We will show that h must be an integer. Let the height h = N/D for positive integers N and D. Since h > 6,
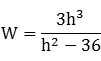
[3]
and we can write [3] as
D < N/6, [4]
Each factor in the denominator divides the numerator. Since D < N by [4], then if D divides N3, it also divides N and h is an integer. Otherwise, D divides 3. If D = 1, then h is again an integer, so assume D = 3. Then [5] becomes
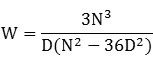
[5]
Since h = N/3 > 6, we have N > 18 and the fraction on the right is a positive integer, so
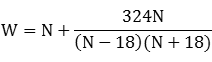
[6]
Each factor of the denominator in [6] divides the numerator (this is a necessary but not sufficient condition for W to be an integer). By long division,
so both N - 18 and N + 18 are factors of 5832, subject to 19 ≤ N ≤ 324:
N ∈ {19,20,21,22,24,26,27,30,36,42,45,54,72,90,99,126,180,234,261} ⋂ {36,54,63,90,144,198,225,306} = {36,54,90}Evaluating in [3], the only solution for which W is an integer is h = 12. From [2], the corresponding value for r2 ish = N/3 ∈ {12,18,30}
so r is irrational. In this case, the volume and surface area are 48π. That bicone is a solid of revolution of a non-equable isosceles triangle with base 12 and height
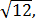 having perimeter
having perimeter
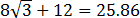 and area
and area

Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.